Circle Theorems

Related Pages
Angles In A Circle
Tangents Of Circles And Angles
Circles
More Lessons for GCSE Maths
Math Worksheets

Circle Theorems Summary

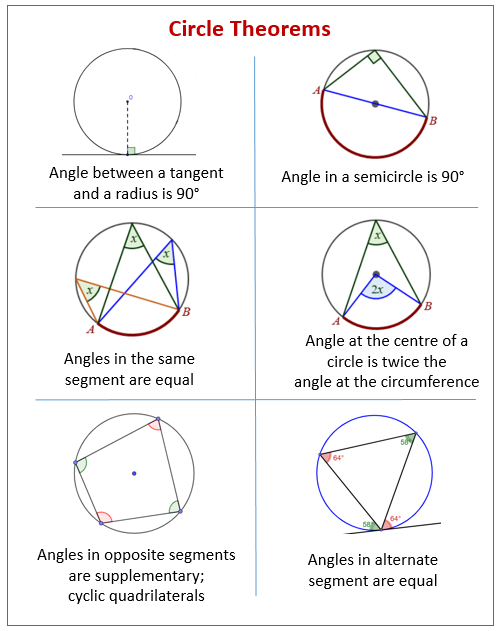
• Angle subtended at the centre of a circle is twice the angle at the circumference.
• The angle between a radius and a tangent is 90 degrees.
• The angle at the centre is twice the angle at the circumference.
• Angles in the same segment are equal.
• The angle in a semi-circle is always 90 degrees.
• The opposite angles in a cyclic quadrilateral always add up to 180 degrees.
• The angle between a circle and a tangent is equal to the angle in the alternate segment.
• The lengths from where two tangents touch a circle to where they meet each other are equal.

The following diagram shows some circle theorems: angle in a semicircle, angle between tangent and radius of a circle, angle at the centre of a circle is twice the angle at the circumference, angles in the same segment are equal, angles in opposite segments are supplementary; cyclic quadrilaterals and alternate segment theorem. Scroll down the page for more examples and solutions of circle theorems.

Circle Theorem Basic definitions
Chord, segment, sector, tangent, cyclic quadrilateral.
Theorem: Angle subtended at the centre of a circle is twice the angle at the circumference.

• Show Video Lesson

Circle Theorems part 1 of 2
The angle between a radius and a tangent is 90 degrees.
The angle at the centre is twice the angle at the circumference.
Angles in the same segment are equal.
The angle in a semi-circle is always 90 degrees.
The opposite angles in a cyclic quadrilateral always add up to 180 degrees.

• Show Video Lesson

Circle Theorems part 2 of 2
The angle between a circle and a tangent is equal to the angle in the alternate segment.
The lengths from where two tangents touch a circle to where they meet each other are equal.

• Show Video Lesson

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.