Derivatives of Exponential Functions

Related Topics:
More Lessons for Calculus
Math Worksheets



The function f(x) = 2^x is called an exponential function because the variable x is the variable. Do not confuse it with the function g(x) = x², in which the variable is the base

The following diagram shows the derivatives of exponential functions. Scroll down the page for more examples and solutions on how to use the derivatives of exponential functions.

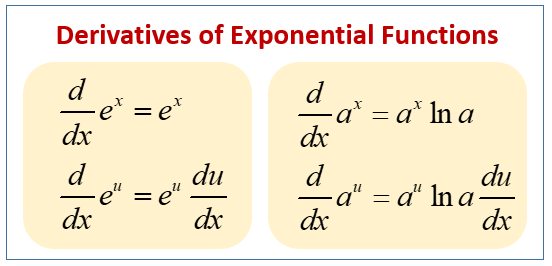
In general, an exponential function is of the form

f(x) = a^x where a is a positive constant.

Derivative of the Natural Exponential Function

The exponential function f(x) = e^x has the property that it is its own derivative. This means that the slope of a tangent line to the curve y = e^x at any point is equal to the y-coordinate of the point.

We can combine the above formula with the chain rule to get

Example:

Differentiate the function y = e ^{sin x}

Solution:

Example:

Differentiate the function y = e^–3xsin4x

Solution:

Using the Product Rule and the above formulas, we get

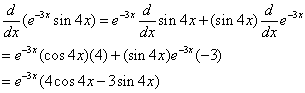
Derivative of a^x

Derivative of a^g(x)

Example:

Differentiate y = x³ + 3^x

Solution:

Example:

Differentiate y = 5^2x+1

Solution:

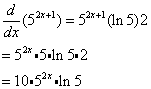
Derivatives of Exponential Functions
The derivative of an exponential function can be derived using the definition of the derivative. Derivatives of exponential functions involve the natural logarithm function, which itself is an important limit in Calculus, as well as the initial exponential function. The derivative is the natural logarithm of the base times the original function.
Derivatives of Exponential Functions with Base e

• Show Step-by-step Solutions

Exponential Functions and Derivatives
This video gives the formula to find derivatives of exponential functions and does a few examples of finding derivatives of exponential functions.

• Show Step-by-step Solutions

Rotate to landscape screen format on a mobile phone or small tablet to use the Mathway widget, a free math problem solver that answers your questions with step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.