Rate, Time, Distance - Algebra Word Problems
In these lessons, we will learn about rate, time, distance word problems and how to solve “motion” word problems.
Related Pages
Rate, Time, Distance Word Problems
Distance Problems
Distance Word Problems
Average Speed Problems
Motion Word Problems: Rate, Time, Distance
The following diagram shows how to solve Rate-Time-Distance Problems. Scroll down the page for more examples and solutions on how to solve Rate-Time-Distance Problems or Motion Problems.
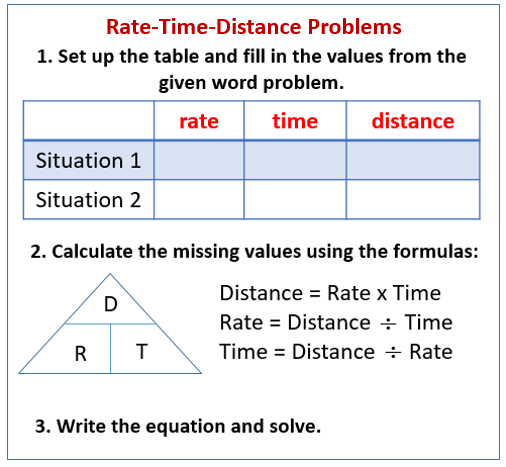
Students learn to solve introductory “motion” word problems - for example, cars traveling in opposite directions, bikers traveling toward each other, or one plane overtaking another. Students should first draw a diagram to represent the relationship between the distances involved in the problem, then set up a chart based on the formula rate times time = distance. The chart is then used to set up the equation.
Example: Two cars leave from the same place at the same time and travel in opposite directions. One car travels at 55 mph and the other at 75 mph. After how many hours will they be 520 miles apart?
Algebra Word Problem: Distance Rate and Time
Appropriate for a student in Algebra 1 or Algebra 2
Example: Two private jets start from Chicago and travel in opposite directions. The speed of the first jet is ten less than two times the speed of the second jet. In 3 hours they are 105 miles apart. Find the speed of each jet.
Algebra & Rate, Time, Distance Problems
Examples:
- A passenger train travels ate 80 mph. A freight train travels at 30 mph. If they travel in opposite directions. How long will it take before they are 275 miles apart?
- Sue can ride her bike 12 miles per hour faster than Mary can walk. Sue rides 95 miles in the same time Mary walks 35 miles. What is each person’s rate?
Distance, Rate, Time Word Problems
Examples:
An object is in uniform motion when it moves without changing its speed, or rate. The examples illustrate
three types of problems involving uniform motion. Each is solved by using a chart, a sketch, and the
distance formula.
- Lea left home and drove toward the ferry office at an average speed of 22 km/h. Trevon left at the same time and drove in the opposite direction with an average speed of 43 km/h. How long does Trevon need to drive before they are 65 km apart?
- Maria left home and traveled toward the capital at the average speed of 80 km./h. Some time later Imani left traveling in the opposite direction with the average speed of 45 km/h. After Maria had traveled for six hours they were 705 km apart. Find the number of hours Imani traveled.
- A passenger train traveled to the repair yards and back. On the trip there it traveled 68 mph and on the return trip it went 85 mph. How long did the trio there take if the return trip took four hours?
- A submarine traveled to St. Vincent and back. It took three hours less time to get there than it did to get back. The average speed on the trip there was 30 km/h. The average speed on the way back was 20 km/h. How many hours did the trip there take?
- Micaela left the hardware store and traveled toward her friend’s house at an average speed of 25 km/h. Nicole left some them later traveling in the same direction at an average speed of 30 km/h. After traveling for five hours, Nicole caught up with Micaela. How long did Macaela travel before Nicole caught up?
- A passenger plane left New York and flew east. A jet left one hour later flying at 280 km/h in an effort to catch up to the passenger plane. After flying for seven hours, the jet finally caught up. Find the passenger plane’s average speed.
Challenge #1
Julio left school and drove toward the recycling plant at an average speed of 30 km/h. Aliyah left four
hours later and drove in the opposite direction with an average speed of 50 km/h. Find the number of
hours Aliyah needs to drive before they are 280 km apart.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.