Population Mean And Sample Mean
The arithmetic mean is the average of a group of numbers and is computed by summing all numbers and dividing by the number of numbers. The arithmetic mean is also usually just called the mean.
Related Pages
Mean
Center Of A Distribution
Central Tendency
More Statistics Lessons
In these lessons, we will distinguish between the population mean and sample mean.
The following table gives the formula for the population mean and the formula for the sample mean. Scroll down the page for examples and solutions on the differences between the population mean and sample mean and how to use them.
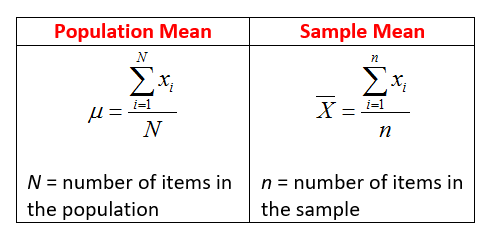
A population is a collection of persons, objects or items of interest.
A sample is a portion of the whole and, if properly taken, is representative of the whole.
The population mean is represented by the Greek letter
mu (μ). It is given by the formula
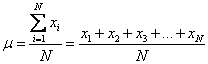
The capital Greek letter sigma (𝚺) is commonly used in mathematics to represent a summation
of all the numbers in a grouping.
N is the number of terms in the population.
The sample mean is represented by x bar
![]() . It is given by
the formula
. It is given by
the formula
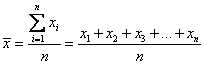
n is the number of terms in the sample.
Sample Mean Versus Population Mean
The following video shows how to find the sample mean and highlights the difference between the mean of a sample and the mean of a population. In statistics, we use data from a random sample to represent the population at large. From that sample mean, we can infer things about the population mean. We infer the population mean from the sample mean because we are not able to collect the data from the entire population.
What Is Population Mean And Sample Mean?
Sample Mean is the mean of sample values collected.
Population Mean is the mean of all the values in the population.
If the sample is random and sample size is large then the sample mean would be a good estimate of the population mean.
How To Calculate A Point Estimate For A Population Mean?
A point estimate is the value of a statistic that estimates the value of a parameter. For example, the sample mean is a point estimate of the population mean.
Arithmetic Mean For Samples And Populations
The arithmetic mean is a single value meant to “sum up” a data set.
To calculate the mean, add up all the values and divide by the number of values.
There are two types of arithmetic mean: population mean (μ) and sample mean (x̄).
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.