Algebra: Isolate A Variable (Transposition)
In these lessons, we will learn one of the basic techniques of simplifying an algebraic equation so that we can eventually solve the equation.
Related Pages
Basic Algebra
Algebra Lesson - Transposition
Combining Like Terms
Solving Equations
More Algebra Lessons
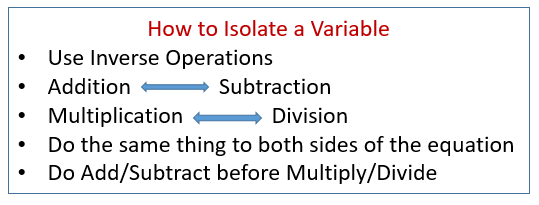
How To Isolate A Variable?
Transposition is a method to isolate the variable to one side of the equation and everything else to the other side so that you can solve the equation.
Two methods are covered here:
- Method 1: Using inverse operations
- Method 2: a shortcut trick that allows you to work faster
The following table gives the steps to Isolate a Variable. Scroll down the page for examples and solutions.
Method 1
A quick review of the basic principles - all equations have two sides: a Left Side (LS) and a Right Side (RS). The common transposition method is to do the same thing (mathematically) to both sides of the equation, with the aim of bringing like terms together and isolate the variable (or the unknown quantity).
For Example:
5x + 8 = 3x – 6
We want to get rid of the number 8 from the left side.
So we subtract 8 from both sides of the equation.
| 5x + 8 | = | 3x – 6 | original equation |
| – 8 | = | – 8 | subtract 8 from both sides |
| 5x | = | 3x – 14 | resulting equation |
Next, we want to get rid of 3x from the right side.
So, we subtract 3x from both sides of the equation.
| 5x | = | 3x – 14 | result from above |
| – 3x | = | – 3x | subtract 3x from both sides |
| 2x | = | – 14 | resulting equation |
Now, we want to get rid of the coefficient 2.
So, we divide 2 from both sides of the equation.
| 2x | = | – 14 | result from above |
| ÷2 | = | ÷2 | divide both sides by 2 |
| x | = | – 7 | resulting solution |
Check the Answer
Now check your answer x = –7 by plugging it back into the original equation.
5x + 8 = 3x – 6
LS: 5 × (– 7) + 8 = – 35 + 8 = – 27
RS: 3 × (– 7) – 6 = – 21 – 6 = – 27
LS = RS, ∴ answer is correct.
Algebra: How to solve equations by isolating the variable?
An equation is a symbolic statement that two algebraic expressions are equal.
To solve an equation means to find the variable or unknown.
The rule is that the same operation must be done on both sides of the equation to preserve equality.
Examples:
- x + 4 = 20
- x - 4 = 20
- 4x = 20
- x/4 = 20
Isolate the variable: - 5x - x = 20 + 4
- 9 - 4x = 8x
- 8 - 5x - x = 14
How to isolate a variable and solve linear equations (variables on both sides of the equation)?
Example:
Solve for y and check your solution
20 - 7y = 6y - 6
How to isolate the variable using inverse operations to solve fraction equations?
Examples:
a + 1/2 = 4
3/7 + n = 2 2/5
5/7 x = 4 2/3
3/4 b = -24
How to isolate a variable in a formula?
- 2p = kx - q, isolate for x
- (3b -4)/2 = C, isolate for b
How to isolate a variable when the variable or expression is in the denominator?
- 8/y = 3
- (x + 3)/(x + 5) = 20
- 5/(x + 3) = 4/(x + 4)
Transposition (Rearranging Equations) - Introduction
What is transposition? What is it used for?
Method 2
In this method we isolate the variable by moving like terms to one side of the equation. To maintain the equality of the equation, when removing a term from one side of the equation we perform the opposite operation to the other side.
For example:
5x + 8 = 3x – 6
To remove + 8 from the LS, we subtract 8 from the RS.
![]()
To remove + 3x from the RS, we subtract 3x from the LS.
![]()
To remove the coefficient 2, we divide 2 on the RS.
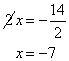
When you have grasped Method 2, it is faster because it allows you to perform several steps at the same time to isolate the variable.
For example:
5x + 8 = 3x – 6
![]()
Shortcut Trick to help you solve equations (Variable on one side)
Examples:
- (3x + 5)/7 = 2
- (5x - 8)/3 = 4
- \(\sqrt {{x^2} + 7} + 5 = 9\)
- \(\frac{{\sqrt {2x} }}{2} = 2\)
- \(\frac{{\sqrt {5x - 6} \times 4}}{2} = 6\)
Shortcut Trick to help you solve equations (Variables on both sides)
Examples:
- 3x + 4 = 2x + 6
- 2x + 1 = 4x - 9
- x - 3 = 4x + 6
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.