Exterior Angles Of A Triangle
Related Pages
Exterior Angle Theorem
Types Of Triangles
Interior Angles of a Triangle
Angles In A Triangle
In these lesson, we will learn
- about exterior angles of triangles
- how to find the unknown exterior angle of a triangle
- how to prove that the sum of exterior angles of a triangle is 360°
The following diagrams give the theorems involving the exterior angles of triangles. Scroll down the page for more examples and solutions.
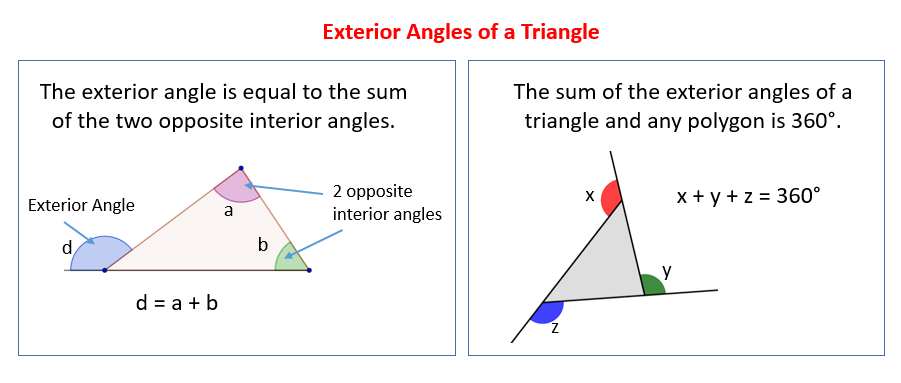
Exterior Angles Of A Triangle
An exterior angle of a triangle is formed by any side of a triangle and the extension of its adjacent side.
The Exterior Angle Theorem states that
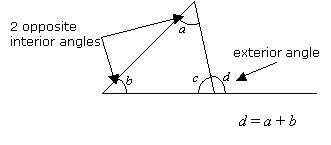
An exterior angle of a triangle is equal to the sum of the two
opposite interior angles.
Example:
Find the values of x and y in the following triangle.
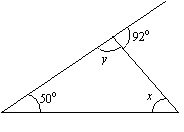
Solution:
x + 50° = 92° (sum of opposite interior angles = exterior angle)
x = 92° – 50° = 42°
y + 92° = 180° (interior angle + adjacent exterior angle = 180°.)
y = 180° – 92° = 88°
What is an exterior angle and how to find the unknown exterior angle of a triangle?
The angles on a straight line add up to 180°.
The interior angles of a triangle add up to 180°
An exterior angle of a triangle is formed when any side is extended outwards.
How to find a missing angle outside of a triangle?
How to define the interior and exterior angles of a triangle and then state several theorems involving the interior and exterior angles of a triangle
The exterior angles of a triangle are the angles that form a linear pair with the interior angles by extending the sides of a triangle.
The sum of the exterior angles of a triangle and any polygon is 360 degrees.
The remote angles are the two angles in a triangle that are not adjacent angles to a specific exterior angle.
The sum of the remote interior angles is equal to the non-adjacent exterior angle.
Sum Of Exterior Angles Of A Triangle
Two column proof of the sum of the exterior angles of a triangle is 360 degrees.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.