Adding Or Subtracting Numbers In Scientific Notation

Scientific Notation: Addition And Subtraction

The following diagram shows how to add or subtract numbers in scientific notation. Scroll down the page for more examples on adding and subtracting with scientific notation.

 

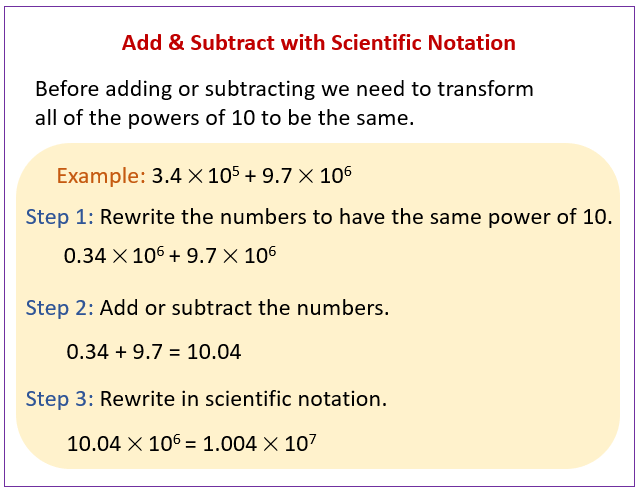
To add or subtract two numbers in scientific notation:
Step 1: Adjust the powers of 10 in the 2 numbers so that they have the same index. (Tip: It is easier to adjust the smaller index to equal the larger index).

Step 2: Add or subtract the numbers.

Step 3: Give the answer in scientific notation.

Example:
Evaluate 2 × 10³ + 3.6 × 10⁴, giving your answer in scientific notation.

Solution:

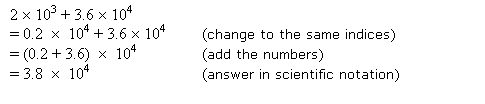
Example:
Evaluate 7 × 10⁵ – 5.2 × 10⁴, giving your answer in scientific notation.

Solution:

Test your knowledge of scientific notations with these five sets of scientific notation worksheets:

• Scientific to Standard Notation
  
• Standard to Scientific Notation
  
• Multiply Scientific Numbers
  
• Divide Scientific Numbers
  
• Add & Subtract Scientific Numbers
  

Add And Subtract Numbers In Scientific Notation With Same Or Different Exponents And Negative Exponents

This video explains how to add and subtract numbers written in scientific notation, whether or not they have the same exponent.

Examples:
(3.769 × 10⁵) + (4.21 × 10⁵)
(8.14 × 10^-2) - (2.01 × 10^-2)
(7.58 × 10⁵) + (2.871 × 10⁶)
(2.9785 × 10^-8) - (5.72 × 10^-10)
(4.86 × 10³) - (4.72 × 10³)

• Show Video Lesson

Why You Have To Make Sure The Exponents Are The Same?

This video explains why when adding and subtracting numbers in scientific notation, you have to make sure the exponents are the same.

Example:
(7.1 × 10³) + (5 × 10²)

• Show Video Lesson

Rules For Scientific Notation

This video reviews scientific notation and shows how to add and subtract in Scientific Notation.

Rule for scientific notation:

• If the exponent goes down, the decimal point goes right.
• If the decimal point goes right, the exponent goes down.
• If the exponent goes up, the decimal goes left.
• If the decimal point goes left, the exponent goes up.

• Show Video Lesson

Scientific Notation - Addition And Subtraction Rules

This video explains how to do add and subtract in scientific notation without the use of a calculator.

• The powers of 10 must be the same in both terms in order to add/subtract.
• Once the power of 10 matches, just add or subtract.
• Adjust the answer to scientific notation.

• Show Video Lesson

Scientific Notation - Adding And Subtracting

Learn how to add and subtract in scientific notation.
Add and subtract numbers in different bases by rewriting their exponents to be the same.

Example:
(3.5 × 10⁴) + (3.1 × 10⁵)

• Show Video Lesson

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.