Nets for 3-Dimensional Shapes
In these lessons, we will learn how to draw and recognize nets for 3-dimensional shapes, especially for students of Grades 5 to 8.
Related Pages
Geometry - Nets Of Solids
3D Nets Animations
More Geometry Lessons
Let’s learn how to represent three-dimensional figures with nets, by analyzing their faces and bases.
A net is a two-dimensional pattern of a three-dimensional figure, that can be folded to form the figure.
We will learn how to represent three-dimensional figures using nets made up of rectangles and triangles, and use the nets to find the surface area of these figures. We will learn how to apply these techniques in the context of solving real-world and mathematical problems.
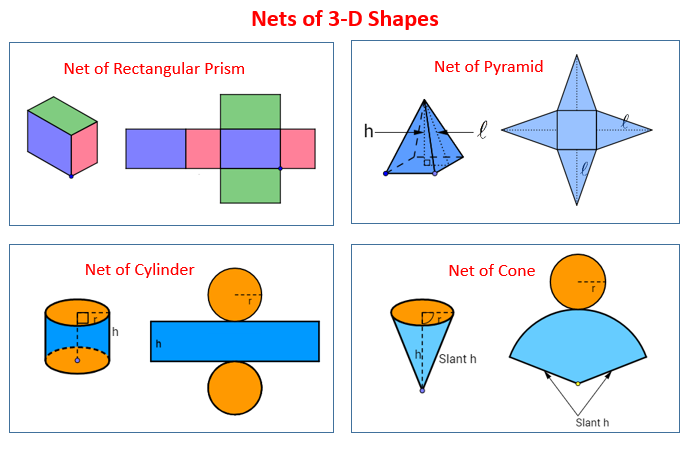
Nets of 3-D shapes: Prisms, Pyramids, Cylinders and Cones
The following diagrams show the nets of some 3d shapes: Prism, Pyramid, Cylinder and Cone. Scroll down the page for more examples and explanations on the net of 3d shapes.
-
Surface Area Worksheets
Printable
Nets of Solid Figures
Find Surface Area using Nets
Surface Area of Rectangular Prisms
Surface Area of Right Prisms
Surface Area of Solids (include composite solids)Online
Surface Area of Cylinder
Surface Area of Sphere
Surface Area of Solids I
Surface Area of Solids II
Surface Area of Solids III
Surface Area of Solids IV
Surface Area of Solids V
Surface Area of Cylinders & Pipes
Surface Area of Cylinders & Spheres
Nets for 3-Dimensional Figures
Learn to use nets to make 3-dimensional figures.
A net is the pattern made when the surface of a three-dimensional figure is laid out flat showing each face of the figure. A net is folded to make a three-dimensional figure.
3D Figures part 1 - Nothing but nets
Drawing two dimensional nets of three dimensional figures.
How to calculate surface area using nets?
Using nets and the formula for area to calculate the surface area of three dimensional figures.
Net of rectangular prism and cube (animated)
This video demonstrates the creation of a net for a cube. The folding and unfolding will be shown visually.
How to recognize the two-dimensional nets that fold into solids?
Net of rectangular pyramid and triangular prism This video demonstrates the creation of a net for a rectangular pyramid and a triangular prism from the 3-d shapes.
What a Net Is (In Solid Geometry) A net of a solid is a 2-dimensional figure which can be folded into that solid.
This video will show nets of cube, cylinder and cone.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.