Congruent Triangles - Hypotenuse Leg Theorem

Related Topics: More Geometry Lessons

In these lessons, we will learn

• the Hypotenuse-Leg Theorem
• why the Hypotenuse-Leg Theorem is enough to prove triangles congruent
• the proof of the Hypotenuse-Leg Theorem using a two-column proof
• how to prove triangle congruence using the Hypotenuse-Leg Theorem

The following diagram shows the Hypotenuse Leg Theorem. Scroll down the page for more examples and solutions of how to use the Hypotenuse Leg Theorem.

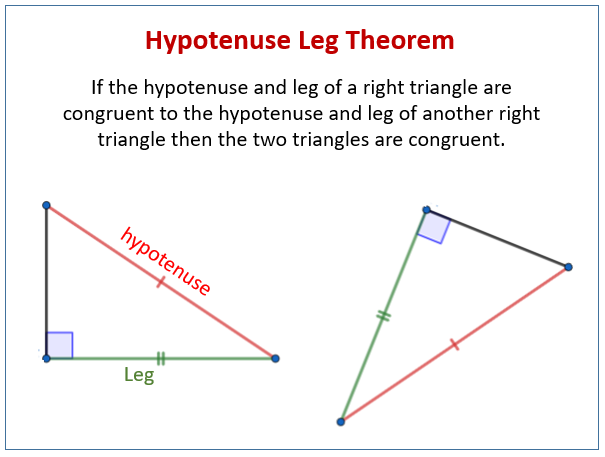
Hypotenuse Leg Theorem

Hypotenuse Leg Theorem is used to prove whether a given set of right triangles are congruent. 

The Hypotenuse Leg (HL) Theorem states that

If the hypotenuse and one leg of a right triangle are equal to the hypotenuse and one leg of another right triangle, then the two right triangles are congruent.

In the following right triangles ΔABC and ΔPQR , if AB = PR, AC = QR then ΔABC ≡ ΔRPQ .

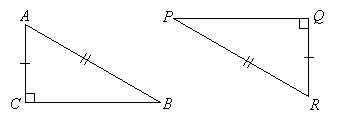
Example:

State whether the following pair of triangles are congruent. If so, state the triangle congruence and the postulate that is used.

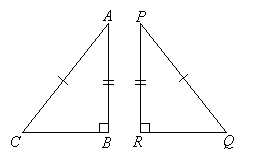
Solution:

From the diagram, we can see that

• ΔABC and ΔPQR are right triangles

• AC = PQ (hypotenuse)
• AB = PR (leg)

So, triangle ABC and triangle PQR are congruent by the Hypotenuse Leg Theorem.

Hypotenuse - Leg Congruence Theorem

The hypotenuse-leg congruence theorem states that if the hypotenuse and leg of one right triangle are congruent to the hypotenuse and leg of another right triangle, the two triangles are congruent.
Explains why HL is enough to prove two right triangles are congruent using the Pythagorean Theorem

• Show Step-by-step Solutions

Examples of the Hypotenuse Leg (HL) Theorem and the Angle-Angle-Side (AAS) Theorem
State whether or not the following pairs of triangles must be congruent. If so, state the triangle congruence and name the postulate that is used.

• Show Step-by-step Solutions

Prove Triangle Congruence with HL Postulate

HL Postulate (Lesson)
A lesson and proof of the HL (Hypotenuse-Leg) postulate using a two-column proof

• Show Step-by-step Solutions

HL Postulate (Practice)

Practice problems and proofs using the HL (Hypotenuse-Leg) Postulate

• Show Step-by-step Solutions

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.