Antiderivative
In these lessons, you will learn the definition of antiderivative, the formula for the antiderivatives of powers of x and the formulas for the antiderivatives of trigonometric functions.
Related Pages
Integral Calculus
Calculus: Integration
Calculus: Derivatives
Calculus Lessons
Definition Of Antiderivative
A function F is called an antiderivative of f on an interval I if F’(x) = f(x) for all x in I.
Formula For The Antiderivatives Of Powers Of x
The general antiderivative of f(x) = xn is
![]()
where c is an arbitrary constant.
Example:
Find the most general derivative of the function f(x) = x–3
Solution:
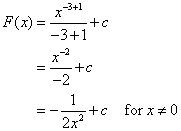
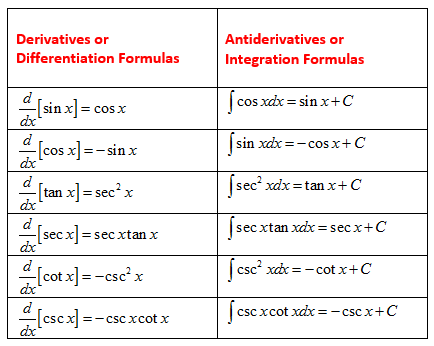
Formulas For The Derivatives And Antiderivatives Of Trigonometric Functions
The tables shows the derivatives and antiderivatives of trig functions. Scroll down the page for more examples and solutions on how to use the formulas.
Example:
Find antiderivative of the function
![]()
Solution:
Rewrite the given function as follows:
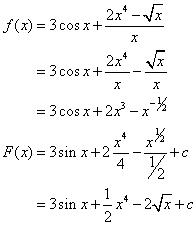
What is the Antiderivative?
The reverse of differentiating is antidifferentiating, and the result is called an antiderivative.
A function F(x) is an antiderivative of f on an interval I if F'(x) = f(x) for all x in I.
You can represent the entire family of antiderivatives of a function by adding a constant to a known antiderivative. So if F(x) is the antiderivative of f(x), then the family of the antiderivatives would be F(x) + C.
What is Integration?
The process of antidifferentiation is often called integration or indefinite integration.
When we find a function’s antiderivative we are actually finding a general solution to a differential equation.
A differential equation in x and y is an equation that involves x, y, and the derivative of y.
What is the Indefinite Integral or Anti-derivative?
An introduction to indefinite integration of polynomials.
Basic Antiderivative Examples
This video has a few examples of finding indefinite integrals of trig functions.
Definition of Antiderivatives
Antiderivatives are the opposite of derivatives. An antiderivative is a function that reverses what the derivative does. One function has many antiderivatives, but they all take the form of a function plus an arbitrary constant. Antiderivatives are a key part of indefinite integrals.
Antidifferentiation
Finding the antiderivatives of a function require a little backwards thinking. Since the derivative of the wanted antiderivative is the given function, checking for correctness is easy. You just take the derivative, and see if it is the given function. Also, antiderivatives of functions happen to be not just one function, but a whole family of functions. This family can be written as a polynomial plus c, where c stands for any constant.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.