

Similarity and Trig Ratios
Related Topics:
Common Core (Geometry)
Common Core for Mathematics
Examples, solutions, videos, and lessons to help High School students learn how to understand that by similarity, side ratios in right triangles are properties of the angles in the triangle, leading to definitions of trigonometric ratios for acute angles.
Common Core: HSG-SRT.C.6
The following diagram shows the connection between trigonometry and similar triangles. Scroll down the page for more examples and solutions for trig ratios and similar triangles.
Using similarity to define sine, cosine, and tangent
Connections between similar triangles and trigonometry.
Trig and similar triangles
Labeling Sides
Learn how to label the sides of a right triangle as 'hypotenuse', 'opposite', and 'adjacent' in relation to one of the triangle's acute angles. Writing Trig Ratios
Writing the basic trig ratios given three side lengths; or given two side lengths. Writing Ratios part 1
Learn how to write the three primary trig. ratios for a right triangle given the three side lengths. Writing Ratios part 2
Learn how to write the three primary trig. ratios for a right triangle given two of the three side lengths. Finding a Side Using Trig
Using the tangent function to find an unknown side in a right triangle - two examples... one where the missing side ends up on the top of the ratio in the trig equation, and another where it ends up on the bottom. Finding an Angle Using Trig
Using the inverse tangent function to find an angle in a right. Trigonometric Ratios on a Calculator
Introduction to the trig function buttons (SIN,COS,TAN), and inverse function buttons (INVERSE SIN,COS,TAN) on a calculator.


Common Core (Geometry)
Common Core for Mathematics
Examples, solutions, videos, and lessons to help High School students learn how to understand that by similarity, side ratios in right triangles are properties of the angles in the triangle, leading to definitions of trigonometric ratios for acute angles.
Common Core: HSG-SRT.C.6
The following diagram shows the connection between trigonometry and similar triangles. Scroll down the page for more examples and solutions for trig ratios and similar triangles.
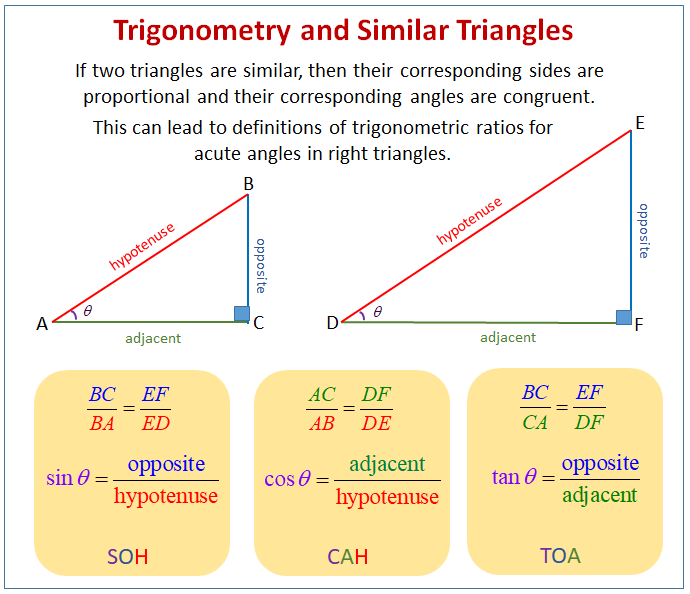
Using similarity to define sine, cosine, and tangent
Connections between similar triangles and trigonometry.
Learn how to label the sides of a right triangle as 'hypotenuse', 'opposite', and 'adjacent' in relation to one of the triangle's acute angles. Writing Trig Ratios
Writing the basic trig ratios given three side lengths; or given two side lengths. Writing Ratios part 1
Learn how to write the three primary trig. ratios for a right triangle given the three side lengths. Writing Ratios part 2
Learn how to write the three primary trig. ratios for a right triangle given two of the three side lengths. Finding a Side Using Trig
Using the tangent function to find an unknown side in a right triangle - two examples... one where the missing side ends up on the top of the ratio in the trig equation, and another where it ends up on the bottom. Finding an Angle Using Trig
Using the inverse tangent function to find an angle in a right. Trigonometric Ratios on a Calculator
Introduction to the trig function buttons (SIN,COS,TAN), and inverse function buttons (INVERSE SIN,COS,TAN) on a calculator.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.



We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.