Rational and Irrational Numbers
Related Pages
Rational Numbers
More Lessons on Numbers
Number Worksheets
GCSE Math Lessons
In these lessons, we will learn about rational and irrational numbers.
How do you know if it is a rational or irrational number?
A rational number is any number that can be expressed as a fraction of two integers.
An irrational number cannot be expressed as a fraction, for example the square root of any number other than square numbers, or a decimal which neither repeats nor terminates. Some examples of irrational numbers are √3, √20, and π(pi).
The following diagram shows some examples of rational numbers and irrational numbers. Scroll down the page for more examples rational and irrational numbers.
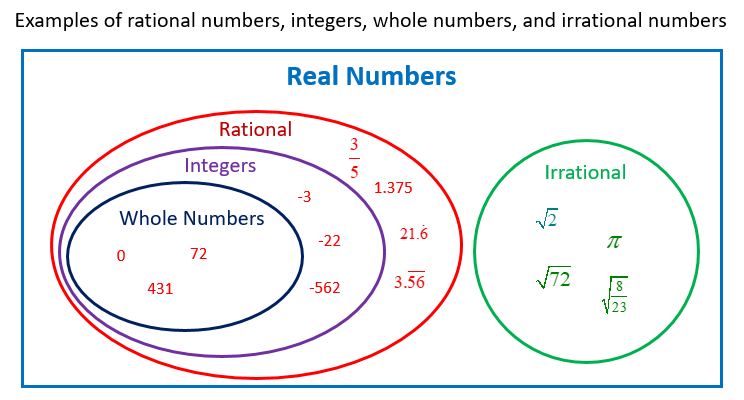
What is the difference between rational and irrational numbers?
This tutorial explains the difference between rational and irrational numbers. Rational and irrational numbers form the Real Numbers.
Rational vs. Irrational Numbers
This video explains the difference between rational and irrational numbers and how to identify rational and irrational numbers.
Irrational Numbers
Although the Greeks initially thought all numeric qualtities could be represented by the ratio of two integers, i.e. rational numbers, we now know that not all numbers are rational. How do we know this?
This video explains why the square root of 2 and the square root of 3 are irrational numbers.
Rational and Irrational Numbers
A review of the difference between rational and irrational numbers and decimals - including square rootes and fraction approximations of pi.
A decimal is irrational if it never terminates and it has no repeating pattern.
Rational Numbers
Three types of rational numbers: Fractions, Decimals and Percents.
Irrational Numbers
Rational and Irrational Numbers
This video defines & compares rational and irrational numbers and give some examples of rational and irrational numbers.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.