Illustrative Mathematics Grade 8, Unit 3, Lesson 9: Slopes Don't Have to be Positive

Related Pages
Illustrative Math
Grade 8

Lesson 9: Slopes Don’t Have to be Positive

Let’s find out what a negative slope means.

Illustrative Math Unit 8.3, Lesson 9 (printable worksheets)

Lesson 9 Summary

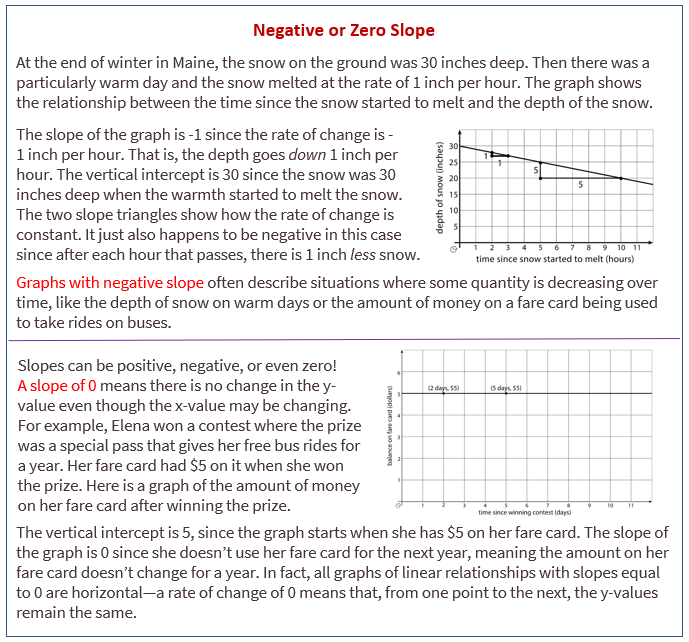
The following diagram shows what a negative or zero slope means.

Lesson 9.1 Which One Doesn’t Belong: Odd Line Out

Which line doesn’t belong?

• See Video for Whole Lesson

Lesson 9.2 Stand Clear of the Closing Doors, Please

Noah put $40 on his fare card. Every time he rides public transportation, $2.50 is subtracted from the amount available on his card.

1. How much money, in dollars, is available on his card after he takes
   a. 0 rides?
   b. 1 ride?
   c. 2 rides?
   d. x rides?
2. Graph the relationship between amount of money on the card and number of rides.

Open Applet

3. How many rides can Noah take before the card runs out of money? Where do you see this number of rides on your graph?

Lesson 9.3 Travel Habits in July

Here is a graph that shows the amount on Han’s fare card for every day of last July. Open Applet

1. Describe what happened with the amount on Han’s fare card in July.
2. Plot and label 3 different points on the line.
3. Write an equation that represents the amount on the card in July, y, after x days.
4. What value makes sense for the slope of the line that represents the amounts on Han’s fare card in July?

Are you ready for more?

Let’s say you have taken out a loan and are paying it back. Which of the following graphs have positive slope and which have negative slope?

1. Amount paid on the vertical axis and time since payments started on the horizontal axis.
2. Amount owed on the vertical axis and time remaining until the loan is paid off on the horizontal axis.
3. Amount paid on the vertical axis and time remaining until the loan is paid off on the horizontal axis.

Lesson 9.4 Payback Plan

Elena borrowed some money from her brother. She pays him back by giving him the same amount every week. The graph shows how much she owes after each week.
Answer and explain your reasoning for each question.

1. What is the slope of the line?
2. Explain how you know whether the slope is positive or negative.
3. What does the slope represent in this situation?
4. How much did Elena borrow?
5. How much time will it take for Elena to pay back all the money she borrowed?

Lesson 9 Practice Problems

1. Suppose that during its flight, the elevation e (in feet) of a certain airplane and its time t, in minutes since takeoff, are related by a linear equation. Consider the graph of this equation, with time represented on the horizontal axis and elevation on the vertical axis. For each situation, decide if the slope is positive, zero, or negative.
   a. The plane is cruising at an altitude of 37,000 feet above sea level.
   b. The plane is descending at rate of 1000 feet per minute.
   c. The plane is ascending at a rate of 2000 feet per minute.
2. A group of hikers park their car at a trail head and hike into the forest to a campsite. The next morning, they head out on a hike from their campsite walking at a steady rate. The graph shows their distance in miles, d, from the car on the day of their hike after h hours.
   a. How far is the campsite from their car? Explain how you know.
   b. Write an equation that describes the relationship between d and h.
   c. After how many hours will the hikers be 16 miles from their car? Explain or show your reasoning.
3. Elena’s aunt pays her $1 for each call she makes to let people know about her aunt’s new business. The table shows how much money Diego receives for washing windows for his neighbors.
   Select all the statements about the situation that are true.
   A. Elena makes more money for making 10 calls than Diego makes for washing 10 windows.
   B. Diego makes more money for washing each window than Elena makes for making each call.
   C. Elena makes the same amount of money for 20 calls as Diego makes for 18 windows.
   D. Diego needs to wash 35 windows to make as much money as Elena makes for 40 calls.
   E. The equation y = 9/10 x, where y is number of dollars and x is number of windows, represents Diego’s situation.
   F. The equation y = x, where y is the number of dollars and x is the number of calls, represents Elena’s situation.
   
4. Each square on a grid represents 1 unit on each side. Match the numbers with the slopes of the lines.

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.