Geometric Constructions Online
Related Topics:
More Lessons for High School Geometry
More Lessons for Geometry
Math Worksheets
A series of free, online High School Geometry Lessons and solutions.
Examples, solutions, videos, worksheets, and activities to help Geometry students.
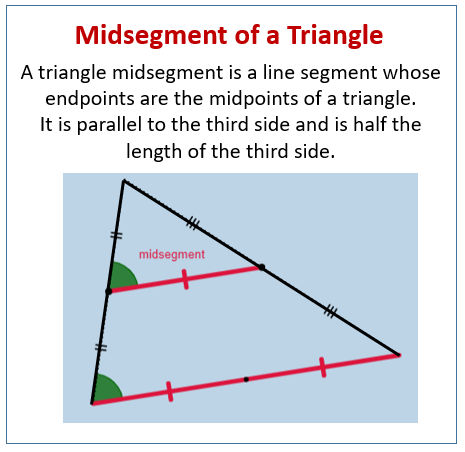
The following diagram shows the midsegment of a triangle. Scroll down the page for more examples and solutions of geometric construction.
In these lessons, we will learn
- how to construct a triangle midsegment Click here
- how to identify the four main points of concurrency in triangles: Incenter, orthocenter, circumcenter, centroid Click here
- how to define circumscribed and inscribed circles and polygons Click here
- how to construct the incenter of a triangle Click here
Constructing a Triangle Midsegment
A triangle mid segment is a line segment whose endpoints are the midpoints of a triangle. The triangle mid segment is parallel to the third side (the side that does not contain an endpoint). Also, the mid-segment is half the length of the third side.
How to define a midsegment in a triangle?
Point of Concurrency
A point of concurrency is where three or more lines intersect in one place. Incredibly, the three angle bisectors, medians, perpendicular bisectors, and altitudes are concurrent in every triangle. There are four types important to the study of triangles: for angle bisectors, the incenter; for perpendicular bisectors, the orthocenter; for the altitudes, the circumcenter; for medians, the centroid.
How to define point of concurrency; how to identify the four main points of concurrency in triangles?
Circumscribed and Inscribed Circles and Polygons
A circle is circumscribed about a polygon if the polygon’s vertices are on the circle. For triangles, the center of this circle is the circumcenter. A circle is inscribed a polygon if the sides of the polygon are tangential to the circle. For triangles, the center of this circle is the incenter. Circumscribed and inscribed circles show up a lot in area problems.
A lesson on polygons inscribed in and circumscribed about a circle.
A review of practice problems involving polygons inscribed in and circumscribed about a circle
This video provides the steps to constructing a circle that is circumscribed about a triangle.
Constructing the Incenter
The point of concurrency of the three angle bisectors of a triangle is the incenter. It is the center of the circle that can be inscribed in the triangle, making the incenter equidistant from the three sides of the triangle. To construct the incenter, first construct the three angle bisectors; the point where they all intersect is the incenter. The incenter is always located within the triangle.
How to construct the incenter using a compass and straightedge?
How to construct an incenter and inscribed circle using a compass and straight-edge?
With three angle bisectors, you construct an Incenter.
An incenter is the center of a circle which can be drawn inscribed inside a triangle (just barely touching the three sides).
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.