Applications of Quadratic Equations
A series of free Basic Algebra Lessons.
These lessons look at the applications of quadratic equations.
Related Pages
Quadratic Equations Word Problems
Solving Quadratic Equations by Factoring
More Lessons for Basic Algebra
Math Worksheets
How to find the vertex and axis of symmetry of a quadratic equation or quadratic function?
How to solve word problems using quadratic equations?
The following diagrams show how to find the vertex of a quadratic function and use that to convert from the general form to the vertex form. Scroll down the page for more examples and solutions for quadratic equations in vertex form.
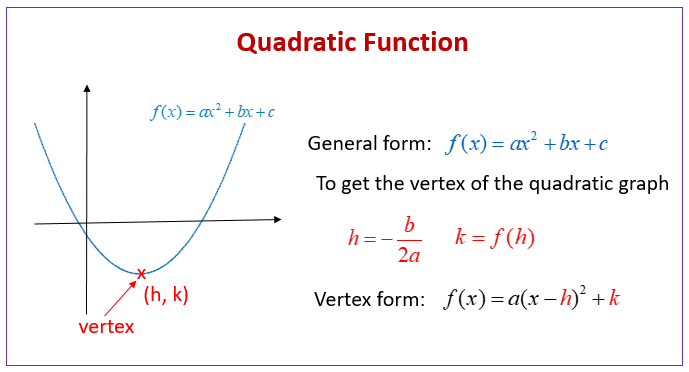
The Vertex and Axis of Symmetry
In a parabola, the vertex is the highest or lowest point on a parabola.
To calculate the vertex of a parabola, we can first calculate the x-value by dividing the opposite of the “b” value by 2 times the “a” value. The vertex is the highest point if the parabola opens downward and the lowest point if the parabola opens upward.
The axis of symmetry is the line that cuts the parabola into 2 matching halves and the vertex lies on the axis of symmetry.
How to find find the vertex, axis of symmetry, domain and range, and x and y intercepts for a quadratic function algebraically?
Example:
Given f(x) = -4x2 + 10x + 9
Find the following
a) vertex
b) axis of symmetry
c) domain and range
d) x-intercepts
e) y-intercepts
Finding the Vertex and Axis of Symmetry
Example:
Find algebraically the equation of the axis of symmetry and the coordinates of the vertex of the parabola whose equation is y = -2x2 - 8x + 3
Applications of Quadratic Equations
In Algebra I and Algebra II, we sometimes need to solve word problems using quadratic equations. When solving word problems, some common quadratic equation applications include projectile motion problems and Geometry area problems. The most important thing when solving these types of problems is to make sure that they are set up correctly so we can use the quadratic equation to easily solve them.
How to solve a quadratic word problem that involves the area of a rectangle?
Example:
Suppose the area of a rectangle is 114.4 m2 and the length is 14 m longer than the width. Find the length and width of the rectangle.
Solving Word Problems involving Distance, Rate, and Time Using Quadratics
Example:
Suppose Jessica drove 100 miles and then increased her speed by 30 mph for the following 200 miles. If the second part took 1 hour less than the first part, what was her average speed?
Solving projectile problems with quadratic equations
Example:
Suppose a projectile is launched from a tower into the air with an initial velocity of 48 feet per second. Its height, h, in feet, above the ground is modeled by the function
h = -16t2 + v0t + 64
where t is the time, in second, since the projectile was launched and v0 is the initial velocity
How long was the projectile in the air?
When did it reach its maximum height?
What was its maximum height?
Application Problem with Quadratic Formula (Projectile Problem)
A ball is shot into the air from the edge of a building 50 feet above the ground. Its initial velocity is 20 feet per second.
The equation is h = -16t2 + 20t + 50 can be used to model the height of the ball after t seconds. About how long does it take for the ball to hit the ground?
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.