Solving Two-Step Equations

Related Pages
Solving Equations
Algebraic Expressions
More Algebra Lessons

The following figure shows how to solve two-step equations. Scroll down the page for more examples and solutions.

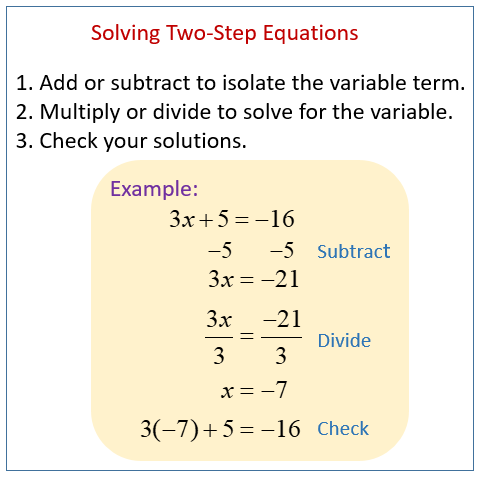
In order to solve two-step equations, we need to work backwards with regards to the order of operations. We would perform the addition & subtraction before multiplication & division.

1. Add or subtract to isolate the variable term.
2. Multiply or divide to solve for the variable.
3. Check your solutions.

Example:
Solve 3x – 2 = 16

Solution:
In the equation 3x – 2 = 16, notice that the variable is being multiplied and has a term being subtracted. To isolate the variable, we need to add 2 to both sides and then divide both sides by 3.

3x – 2 = 16
3x – 2 + 2 = 16 + 2 (add 2 to both sides)
3x = 18 (simplify)
x = 6 (divide both sides by 3)

Check:
3x – 2 = 16
3 • 6 – 2 = 16 (Substitute x = 6 into the original equation)

Example:
Solve

Solution:
Consider the equation, . To isolate the variable, we need to subtract 2 from both sides and then multiply both sides by 4.

Check:

How To Solve Basic Two Step Equations?

Examples:
a) 2x + 3 = 9
b) 5x – 8 = 22
c) 9 – 2x = 23
d) –11 = 4x – 43
e) –15 = 5 – 5x

• Show Video Lesson

How To Solve Two Step Linear Equations With Fractions?

In this video, the equations are solve by working with the fraction, not be clearing the fractions from the equation.

Examples:

1. 2x + 1/4 = 3/5
2. 2/5 x - 1/2 = 3/5
3. 1/4 = 3/5 x - 5
4. 2 1/5 = 2/3 - 1/2 x

• Show Video Lesson

How To Solve Two Step Linear Equations Involving Decimals?

Examples:

1. 0.3x - 2.16 = 9.9
2. x/2.1 + 4.93 = 10.45

• Show Video Lesson

How To Solve The Different Types Of Two Step Equations?

Examples:

1. 2x + 3 = 25
2. x/4 - 2 = -5
3. 4 - 2x = 10
4. 4 = 6 - x/3
5. 4.3 = 1.5x - 5
6. x/3 - 3/4 = 1/6

• Show Video Lesson

Examples Of Solving Two-Step Equations

1. b/3 + 13 = 11
2. 9g + 11 = 2
3. t/4 - 10 = -6
4. -a + 6 = 8
5. 15x + 3 = 48

• Show Video Lesson

How To Solve A Two-Step Equation? (undo PEMDAS in reverse to solve)

Example:
4x + 8 = 24

• Show Video Lesson

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.