Illustrative Mathematics Grade 8, Unit 5, Lesson 21: Cylinders, Cones, and Spheres

Related Pages
Illustrative Math
Grade 8

Lesson 21: Cylinders, Cones, and Spheres

Let’s find the volume of shapes.

Illustrative Math Unit 8.5, Lesson 21 (printable worksheets)

Lesson 21 Summary

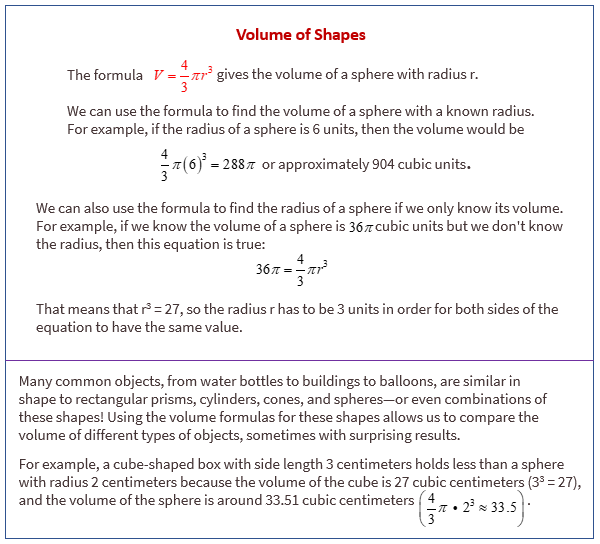
The following diagram shows how to find the radius of a sphere if I know its volume.

Lesson 21.1 Sphere Arguments

Four students each calculated the volume of a sphere with a radius of 9 centimeters and they got four different answers.

• Han thinks it is 108 cubic centimeters.
• Jada got 180π cubic centimeters.
• Tyler calculated 972 cubic centimeters.
• Mai says it is 972π cubic centimeters. Do you agree with any of them? Explain your reasoning.

• See Video for Whole Lesson

Lesson 21.2 Sphere’s Radius

The volume of this sphere with radius r is V = 288π. This statement is true: 288π = 4/3r³π What is the value of r for this sphere? Explain how you know.

Lesson 21.3 Info Gap: Unknown Dimensions

Your teacher will give you either a problem card or a data card. Do not show or read your card to your partner.
If your teacher gives you the problem card:

1. Silently read your card and think about what information you need to answer the question.
2. Ask your partner for the specific information that you need.
3. Explain to your partner how you are using the information to solve the problem.
4. Solve the problem and explain your reasoning to your partner.
   If your teacher gives you the data card:
5. Silently read the information on your card.
6. Ask your partner “What specific information do you need?” and wait for your partner to ask for information. Only give information that is on your card. (Do not figure out anything for your partner!)
7. Before telling your partner the information, ask “Why do you need that information?”
8. After your partner solves the problem, ask them to explain their reasoning and listen to their explanation.
   Pause here so your teacher can review your work. Ask your teacher for a new set of cards and repeat the activity, trading roles with your partner.

Are you ready for more?

A thirsty crow wants to raise the level of water in a cylindrical container so that it can reach the water with its beak.
The container has diameter of 2 inches and a height of 9 inches.
The water level is currently at 6 inches.
The crow can reach the water if it is 1 inch from the top of the container.
In order to raise the water level, the crow puts spherical pebbles in the container. If the pebbles are approximately 1/2 inch in diameter, what is the fewest number of pebbles the crow needs to drop into the container in order to reach the water?

• Show Answers

  Volume of water that needs to be displaced = π(1)²2 = 2π
  Volume of 1 pebble = 4/3 π (1/4)³ = π/48
  
  Number of pebbles = 2π ÷ π/48 = 96

Lesson 20 Practice Problems

1. A scoop of ice cream has a 1.5 inch radius. How tall should the ice cream cone of the same radius be in order to contain all of the ice cream inside the cone?
2. Calculate the volume of the following shapes with the given information. For the first three questions, give each answer both in terms of π and by using 3.14 to approximate π. Make sure to include units.
   a. Sphere with a diameter of 6 inches
   b. Cylinder with a height of 6 inches and a diameter of 6 inches
   c. Cone with a height of 6 inches and a radius of 3 inches
   d. How are these three volumes related?
3. A coin-operated bouncy ball dispenser has a large glass sphere that holds many spherical balls. The large glass sphere has a radius of 9 inches. Each bouncy ball has radius of 1 inch and sits inside the dispenser.
   If there are 243 bouncy balls in the large glass sphere, what proportion of the large glass sphere’s volume is taken up by bouncy balls? Explain how you know.
4. A farmer has a water tank for cows in the shape of a cylinder with radius of 7 ft and a height of 3 ft. The tank comes equipped with a sensor to alert the farmer to fill it up when the water falls to 20% capacity. What is the volume of the tank be when the sensor turns on?

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.