

Sine Ratio Problems
Related Topics: More Trigonometry Lessons
In these lessons, we will learn how to find the angles and sides using the sine ratio and how to solve word problems using the sine ratio.
These two videos show you how to find the missing length of a triangle using the sine function.
We are given a right triangle and two side lengths and a missing length.
We show a right triangle and label the hypotenuse, and two legs the opposite side and adjacent side according to their relation to θ.
Trigonometry Basics : SOHCAHTOA
How to find missing sides using sine, cosine and tangent?
Trig Ratios - SOH - CAH - TOA
Find missing Angle - Using Inverse Sine, Cosine
Example:
A 55ft wire connects a point on the ground to the top of a pole. The cable makes an angle of 60 degrees to the ground. Find the height of the pole to the nearest foot.


In these lessons, we will learn how to find the angles and sides using the sine ratio and how to solve word problems using the sine ratio.
Hints on solving trigonometry problems:
- If no diagram is given, draw one yourself.
- Mark the right angles in the diagram.
- Show the sizes of the other angles and the lengths of any lines that are known
- Mark the angles or sides you have to calculate.
- Consider whether you need to create right triangles by drawing extra lines. For example, divide an isosceles triangle into two congruent right triangles.
- Decide whether you will need Pythagoras theorem, sine, cosine or tangent.
- Check that your answer is reasonable. The hypotenuse is the longest side in a right triangle.
Example:

Calculate the length of the side x, given that sin θ = 0.6
Solution:
![]()
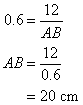
Using Pythagoras’ theorem:
![]()
Find Missing Sides
Find the missing length of a triangle using the sine functionThese two videos show you how to find the missing length of a triangle using the sine function.
We are given a right triangle and two side lengths and a missing length.
We show a right triangle and label the hypotenuse, and two legs the opposite side and adjacent side according to their relation to θ.
How to find missing sides using sine, cosine and tangent?
Find Missing Angles
Trigonometry : using SOHCAHTOA to find missing angles.Trig Ratios - SOH - CAH - TOA
Find missing Angle - Using Inverse Sine, Cosine
Sine Function Word Problems
A word problem involving the trigonometric ratio of sine to calculate the height of a poleExample:
A 55ft wire connects a point on the ground to the top of a pole. The cable makes an angle of 60 degrees to the ground. Find the height of the pole to the nearest foot.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.



We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.