Similarity, Area Ratios and Volume Ratios

Related Pages
More Lessons for Grade 8 Math
Math Worksheets

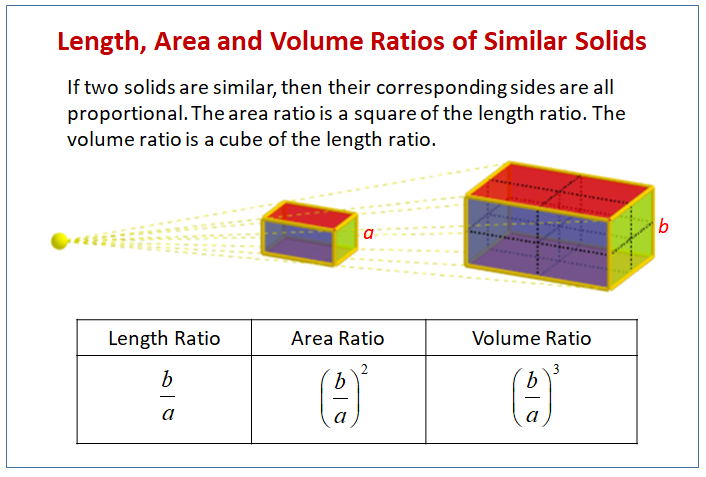
The following diagram shows how to convert between length, area and volume ratios of similar solids. Scroll down the page for more examples and solutions length, area and volume of similar figures.

Similarity and Area Ratios
Is there any relationship between the ratio of the areas of two similar figures?
If two triangles are similar, then their corresponding sides are proportional. Since sides are a length and lengths are one dimensional, the side ratio will not predict the ratio of the areas. To find the area ratios, raise the side length ratio to the second power. This applies because area is a square or two-dimensional property.

Similarity and Volume Ratios
How are the ratios of the surface area of solids related to their corresponding volumes?
If two solids are similar, then their corresponding sides are all proportional. The ratio of their surface areas is the side ratio squared and note that the ratios of the areas does not give the actual surface areas. The volume ratio for the two solids is the side length ratio raised to the third power. Again, this is not the solids' volume, only the ratio of the volumes.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.