Illustrative Mathematics Grade 8, Unit 2, Lesson 7: Similar Polygons

Related Pages
Illustrative Math
Grade 8

Lesson 7: Similar Polygons

Let’s look at sides and angles of similar polygons.

Illustrative Math Unit 8.2, Lesson 7 (printable worksheets)

Lesson 7 Summary

The following diagram shows how to use angle measures and side lengths to conclude that two polygons are not similar.

Lesson 7.1 All, Some, None: Congruence and Similarity

Choose whether each of the statements is true in all cases, in some cases, or in no cases.

1. If two figures are congruent, then they are similar.
2. If two figures are similar, then they are congruent.
3. If an angle is dilated with the center of dilation at its vertex, the angle measure may change.

• See Video for Whole Lesson

Lesson 7.2 Are They Similar?

1. Let’s look at a square and a rhombus.
   Priya says, “These polygons are similar because their side lengths are all the same.” Clare says, “These polygons are not similar because the angles are different.” Do you agree with either Priya or Clare? Explain your reasoning.
2. Now, let’s look at rectangles ABCD and EFGH.
   Jada says, “These rectangles are similar because all of the side lengths differ by 2.” Lin says, “These rectangles are similar. I can dilate AD and BC using a scale factor of 2 and AB and CD using a scale factor of 1.5 to make the rectangles congruent. Then I can use a translation to line up the rectangles.” Do you agree with either Jada or Lin? Explain your reasoning.

Are you ready for more?

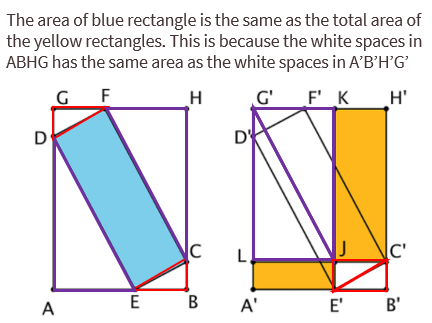
Points A through H are translated to the right to create points A' through H'. All of the following are rectangles: GHBA, FCED, KH’C’J, and LJE’A. Which is greater, the area of blue rectangle DFCE or the total area of yellow rectangles KH’C’J and LJE’A'?

• Show Answer

  

Lesson 7.3 Find Someone Similar

Your teacher will give you a card. Find someone else in the room who has a card with a polygon that is similar but not congruent to yours. When you have found your partner, work with them to explain how you know that the two polygons are similar.

Are you ready for more?

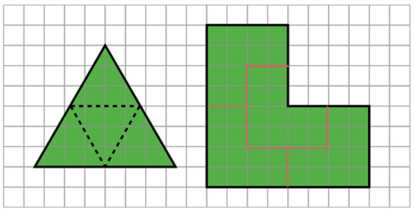
On the left is an equilateral triangle where dashed lines have been added, showing how you can partition an equilateral triangle into smaller similar triangles.
Find a way to do this for the figure on the right, partitioning it into smaller figures which are each similar to that original shape. What’s the fewest number of pieces you can use? The most?

• Show Answer

  

Lesson 7 Practice Problems

1. Triangle DEF is a dilation of triangle ABC with scale factor 2. In triangle ABC, the largest angle measures 82°. What is the largest angle measure in triangle DEF?
2. Draw two polygons that are similar but could be mistaken for not being similar. Explain why they are similar.
3. Draw two polygons that are not similar but could be mistaken for being similar. Explain why they are not similar.
4. These two triangles are similar. Find side lengths and . Note: the two figures are not drawn to scale.
5. Jada claims that B’C’D' is a dilation of BCD using A as the center of dilation.
   What are some ways you can convince Jada that her claim is not true?
6. a. Draw a horizontal line segment AB.
   b. Rotate segment AB 90° counterclockwise around point A. Label any new points.
   c. Rotate segment AB 90° clockwise around point B. Label any new points.
   d. Describe a transformation on segment AB you could use to finish building a square.

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.