Illustrative Mathematics Grade 8, Unit 8, Lesson 3: Rational and Irrational Numbers

Related Pages
Illustrative Math
Grade 8

Lesson 3: Rational and Irrational Numbers

Let’s learn about irrational numbers.

Illustrative Math Unit 8.8, Lesson 3 (printable worksheets)

Lesson 3 Summary

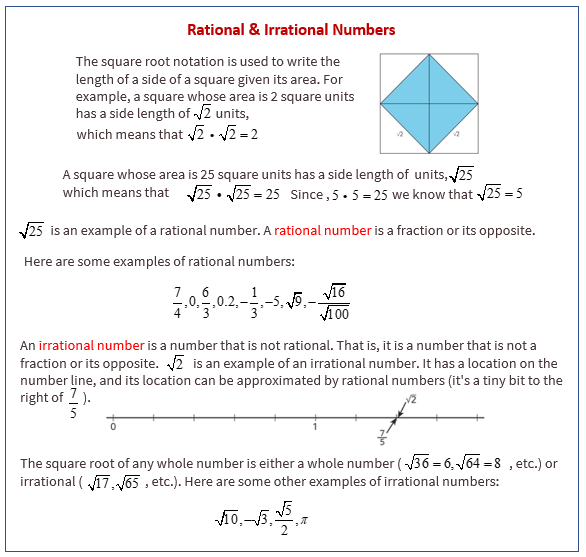
The following diagrams explain and give examples of rational and irrational numbers.

Lesson 3.1 Algebra Talk: Positive Solutions

Find a positive solution to each equation:

• See Video for Whole Lesson

Lesson 3.2 Three Squares

1. Draw 3 squares of different sizes with vertices aligned to the vertices of the grid.
2. For each square:
   a. Label the area.
   b. Label the side length.
   c. Write an equation that shows the relationship between the side length and the area.

Lesson 3.3 Looking for a Solution

Are any of these numbers a solution to the equation x² = 2? Explain your reasoning.

Lesson 3.4 Looking for √2

A rational number is a fraction or its opposite (or any number equivalent to a fraction or its opposite).

1. Find some more rational numbers that are close to √2.
2. Can you find a rational number that is exactly √2?

Are you ready for more?

If you have an older calculator evaluate the expression (577/408)² and it will tell you that the answer is 2, which might lead you to think that √2 = 577/488.

1. Explain why you might be suspicious of the calculator’s result.
   • Show Answer

     √2 is irrational and cannot be written as a fraction.

2. Find an explanation for why 408² · 2 could not possibly equal 577². How does this show that (577/408)² could not equal 2?
   • Show Answer

     408² · 2 must be even.
     577² must be odd because odd · odd = odd
     

3. Repeat these questions for (1414213562375/10000000000000)² ≠ 2, an equation that even many modern calculators and computers will get wrong.
   • Show Answer

     Similarly, 1414213562375² is odd, but 10000000000000 ² · 2 is even.

Lesson 3 Practice Problems

1. Decide whether each number in this list is rational or irrational.
2. Which value is an exact solution of the equation m² = 14?
3. A square has vertices (0,0), (5,2), (3,7), and (-2,5). Which of these statements is true?
4. Rewrite each expression in an equivalent form that uses a single exponent.
5. The graph represents the area of arctic sea ice in square kilometers as a function of the day of the year in 2016.
   a. Give an approximate interval of days when the area of arctic sea ice was decreasing.
   b. On which days was the area of arctic sea ice 12 million square kilometers?
6. The high school is hosting an event for seniors but will also allow some juniors to attend. The principal approved the event for 200 students and decided the number of juniors should be 25% of the number of seniors. How many juniors will be allowed to attend? If you get stuck, try writing two equations that each represent the number of juniors and seniors at the event.

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.