Illustrative Mathematics Grade 8, Unit 8, Lesson 7: A Proof of the Pythagorean Theorem

Related Pages
Illustrative Math
Grade 8

Lesson 7: A Proof of the Pythagorean Theorem

Let’s prove the Pythagorean Theorem.

Illustrative Math Unit 8.8, Lesson 7 (printable worksheets)

Lesson 7 Summary

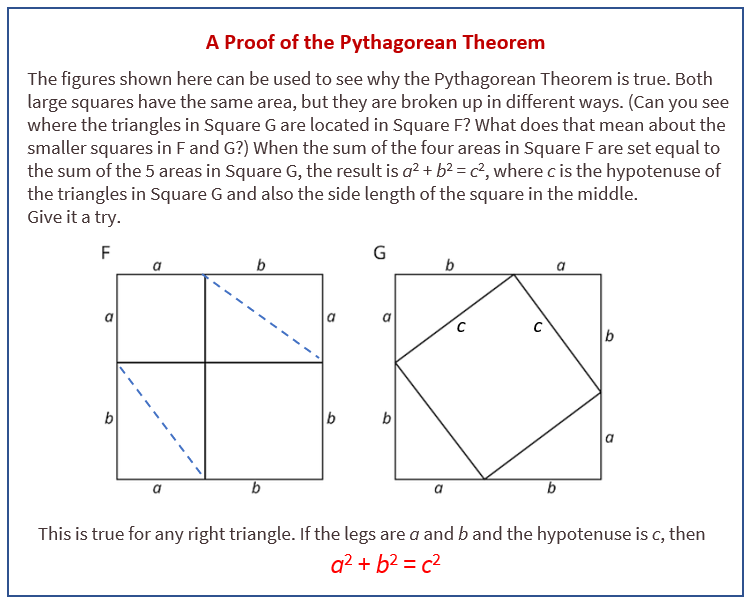
The following diagram gives a proof of the Pythagorean Theorem.

Lesson 7.1 Notice and Wonder: A Square and Four Triangles

What do you notice? What do you wonder?

• See Video for Whole Lesson

Lesson 7.2 Adding Up Areas

Both figures shown here are squares with a side length of a + b. Notice that the first figure is divided into two squares and two rectangles. The second figure is divided into a square and four right triangles with legs of lengths a and b. Let’s call the hypotenuse of these triangles c.

1. What is the total area of each figure?
2. Find the area of each of the 9 smaller regions shown the figures and label them.
3. Add up the area of the four regions in Figure F and set this expression equal to the sum of the areas of the five regions in Figure G. If you rewrite this equation using as few terms as possible, what do you have?

Are you ready for more?

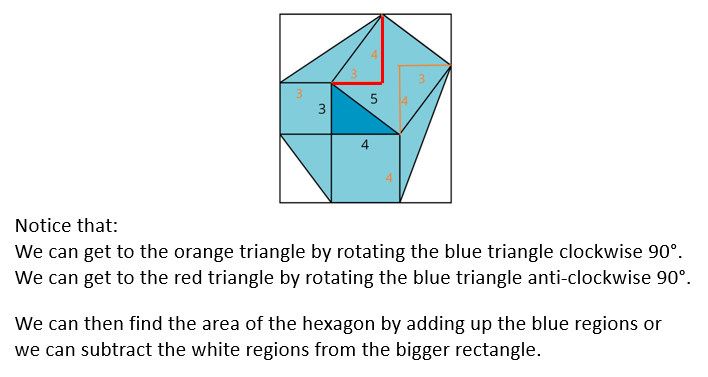
Take a 3-4-5 right triangle, add on the squares of the side lengths, and form a hexagon by connecting vertices of the squares as in the image. What is the area of this hexagon?

• Show Answer

  

Lesson 7.3 Let’s Take It for a Spin

Find the unknown side lengths in these right triangles

Lesson 7.4 A Transformational Proof

Use the applets to explore the relationship between areas.

• Consider Squares A and B.
• Check the box to see the area divided into five pieces with a pair of segments.
• Check the box to see the pieces.
• Arrange the five pieces to fit inside Square C.
• Check the box to see the right triangle.
• Arrange the figures so the squares are adjacent to the sides of the triangle.

1. If the right triangle has legs a and b and hypotenuse c, what have you just demonstrated to be true?
   Open Applet
   
2. Try it again with different squares. Estimate the areas of the new Squares, A, B, and C and explain what you observe.
   Open Applet
   
3. Estimate the areas of these new Squares, A, B, and C, and then explain what you observe as you complete the activity.
   Open Applet
   
4. What do you think we may be able to conclude?

Lesson 7 Practice Problems

1. a. Find the lengths of the unlabeled sides.
   b. One segment is n units long and the other is p units long. Find the value of n and p. (Each small grid square is 1 square unit.)
2. Use the areas of the two identical squares to explain why 5² + 12² = 13² without doing any calculations.
3. Each number is between which two consecutive integers?
4. a. Give an example of a rational number, and explain how you know it is rational.
   b. Give three examples of irrational numbers.
5. Write each expression as a single power of 10.
6. Andre is ordering ribbon to make decorations for a school event. He needs a total of exactly 50.25 meters of blue and green ribbon. Andre needs 50% more blue ribbon than green ribbon for the basic design, plus an extra 6.5 meters of blue ribbon for accents. How much of each color of ribbon does Andre need to order?

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.