Illustrative Mathematics Grade 7, Unit 2, Lesson 11: Interpreting Graphs of Proportional Relationships

Related Pages
Illustrative Math
Grade 7

Lesson 11: Interpreting Graphs of Proportional Relationships

Let’s read stories from the graphs of proportional relationships.

Illustrative Math Unit 7.2, Lesson 11 (printable worksheets)

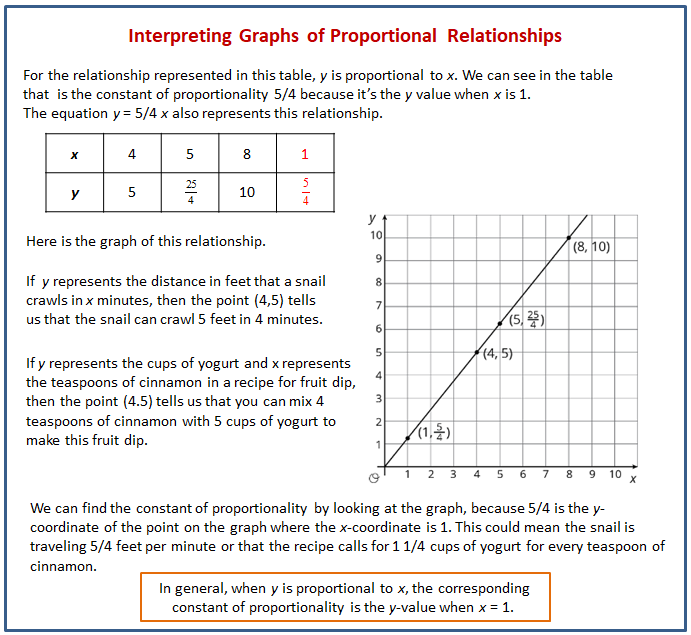
Lesson 11 Summary

The following diagram shows how to find the constant of proportionality from a table or graph.

Lesson 11.1 What Could the Graph Represent?

Here is a graph that represents a proportional relationship.

1. Invent a situation that could be represented by this graph.
2. Label the axes with the quantities in your situation.
3. Give the graph a title.
4. There is a point on the graph. What are its coordinates? What does it represent in your situation?
   

• See Video for Whole Lesson

Lesson 11.2 Tyler’s Walk

Tyler was at the amusement park. He walked at a steady pace from the ticket booth to the bumper cars.

1. The point on the graph shows his arrival at the bumper cars. What do the coordinates of the point tell us about the situation?
2. The table representing Tyler’s walk shows other values of time and distance. Complete the table. Next, plot the pairs of values on the grid.
3. What does the point (0,0) mean in this situation?
4. How far away from the ticket booth was Tyler after 1 second? Label the point on the graph that shows this information with its coordinates.
5. What is the constant of proportionality for the relationship between time and distance? What does it tell you about Tyler’s walk? Where do you see it in the graph?
   

Are you ready for more?

1. If Tyler wanted to get to the bumper cars in half the time, how would the graph representing his walk change? How would the table change? What about the constant of proportionality?

Lesson 11.3 Seagulls Eat What?

4 seagulls ate 10 pounds of garbage. Assume this information describes a proportional relationship.

1. Plot a point that shows the number of seagulls and the amount of garbage they ate.
2. Use a straight edge to draw a line through this point and (0,0).
3. Plot the point (1,k) on the line. What is the value of k? What does the value of k tell you about this context?
   Open Applet

Lesson 11 Practice Problems

1. There is a proportional relationship between the number of months a person has had a streaming movie subscription and the total amount of money they have paid for the subscription. The cost for 6 months is $47.94. The point (6, 47.94) is shown on the graph below.
   a. What is the constant of proportionality in this relationship?
   b. What does the constant of proportionality tell us about the situation?
   c. Add at least three more points to the graph and label them with their coordinates.
   d. Write an equation that represents the relationship between C, the total cost of the subscription, and m, the number of months.
   
2. The graph shows the amounts of almonds, in grams, for different amounts of oats, in cups, in a granola mix. Label the point (1,k) on the graph, find the value of k, and explain its meaning.
   
3. To make a friendship bracelet, some long strings are lined up; then, one string is tied in a knot with each of the other strings to create a row of knots. A new string is chosen and knotted with the all the other strings to create a second row. This process is repeated until there are enough rows to make a bracelet to fit around your friend’s wrist.
   Are the number of knots proportional to the number of rows? Explain your reasoning.
   
4. What information do you need to know to write an equation relating two quantities that have a proportional relationship?
   

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.