Half Angle Identities
Examples, solutions, videos, worksheets, and activities to help PreCalculus students learn how to derive and use the half angle identities.
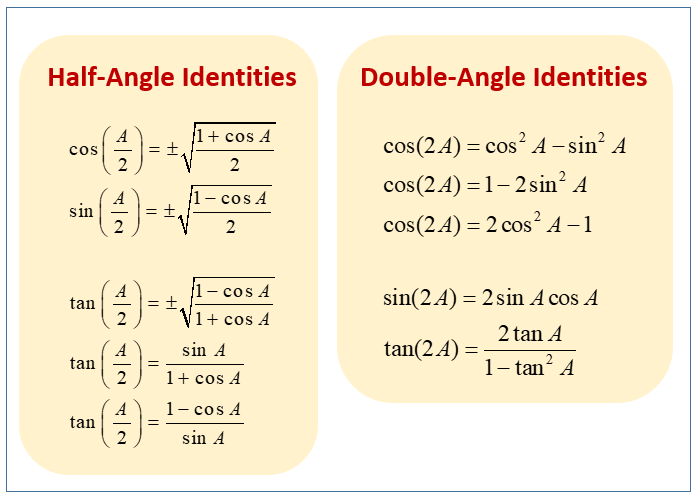
The following diagrams show the half-angle identities and double-angle identities. Scroll down the page for more examples and solutions on how to use the half-angle identities and double-angle identities.
Half Angle Identities to Evaluate Trigonometric Expressions
This video gives some half angle identities and show how they can be used to solve some trigonometric equations.
Example:
Find the exact value of the following:
sin(22.5°)
Half Angle Identities to Evaluate Trigonometric Expressions, Example 2.
Example:
Find the exact value of the following:
tan(105°)
Half Angle Identities to Evaluate Trigonometric Expressions, Example 3.
Example:
Find sin(9/2) if cos a = 3/5 for 9° ≤ a ≤ 90°
Solving Trigonometric Equations using Half-Angle Identities
Example:
Solve tan (x/2) + sin x = 0 for x ∊ [0, 2π}
How half-angle identities can be used to determine function values?
Example:
- Determine the exact value of sin(π/8)
- Determine the exact value of cos(105°)
- Given cos A = -2/3 in quadrant II, determine cos(A/2), sin(A/2) and tan(A/2).
Using half-angle formulas in trigonometry
Example:
Let sin A = 4/5 with A in quadrant II.
Find
- sin (A/2)
- cos 2A
- sec 2A
Derive and use Half-Angle Identities
The derivations of the half-angle identities for both sine and cosine, plus listing the tangent ones. Then a couple of examples using the identities.
Examples:
- Find the exact value for sin (9π/8)
- Find cos x/2 if sin x = -4/5 with 3π/2 < x < 2π
How to derive the half angle trigonometry identities for cosine, sine and tangent?
The Half-Angle Identities
The half angle identities come from the power reduction formulas using the key substitution α = θ/2 twice, once on the left and right sides of the equation. With half angle identities, on the left side, this yields (after a square root) cos(θ/2) or sin(θ/2); on the right side cos(2α) becomes cos(θ) because 2(1/2) = 1. For a problem like sin(pi/12), remember that θ/2 = π/12, or θ = π/6, when substituting into the identity.
How to use the power reduction formulas to derive the half-angle formulas?
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.