Geometric Sequences - nth Term
In these lessons, we will look at geometric sequences and how to find the nth term of a geometric sequence.
Related Pages
Number Sequences
Linear Sequences
Quadratic and Cubic Sequences
The following figure gives the formula for the nth term of a geometric sequence. Scroll down the page for examples and solutions on how to use the formula.
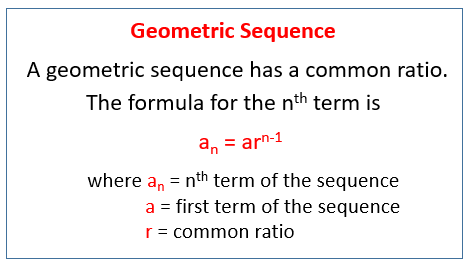
What Is The Formula For A Geometric Sequence?
The formula for a geometric sequence is
an = a1rn - 1 where a1 is the first term and r is
the common ratio.
Geometric Sequences
This video looks at identifying geometric sequences as well as finding the nth term of a geometric sequence.
Example:
- Given a1 = 5, r = 2, what is the 6th term?
- Given a1 = 11, r = -3, what is a8?
Geometric Sequences: A Formula For The nth Term.
This video shows how derive the formula to find the ‘n-th’ term of a geometric sequence by considering an example. The formula is then used to find another term of the sequence.
Finding The nth Term Of A Geometric Sequence
How to find any term of a geometric sequence?
Example:
5, 10, 20, 40, …
Find The Formula For A Geometric Sequence Given Terms
This video explains how to find the formula for the nth term of a given geometric sequence given three terms of the sequence.
Example:
Given the information about the geometric sequence, determine the formula for the nth term.
a0 = 5, a1 = 40/9, a3 = 320/81, …
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.