Gas Laws
In these lessons, we will learn and apply:
- Boyle’s Law
- Charles' Law
- Gay-Lussac’s Law
- Combined Gas Law
- Ideal Gas Law
Related Pages
Solving Gas Law Problems
High School Chemistry
Chemistry Lessons
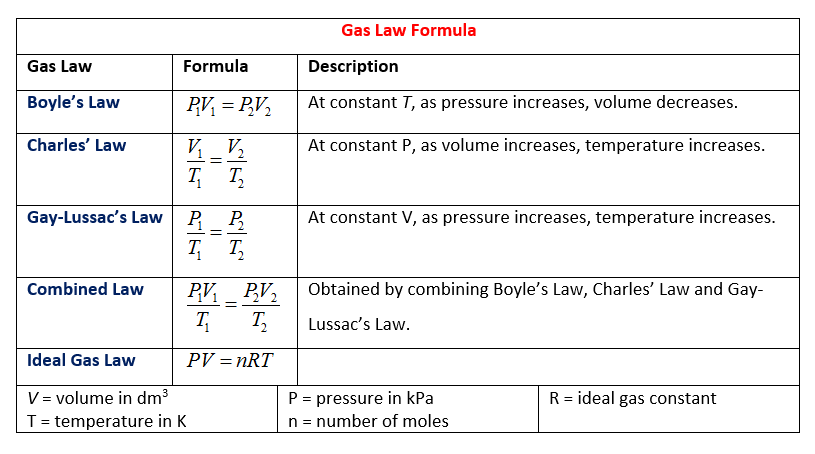
The following table gives the Gas Law Formulas. Scroll down the page for more examples and solutions
on how to use the Boyle’s Law, Charles’Law, Gay-Lussac’s Law, Combined Gas Law and Ideal Gas Law.
Boyle’s Law
Boyle’s Law states that volume of a given amount of gas held at a constant temperature varies inversely the with pressure. The relationship between pressure and volume of Boyle’s Law is expressed in mathematical terms as P1V1= P2V2.
An Introduction To The Relationship Between Pressure And Volume
A lesson on how to solve gas problems with Boyle’s Law.
Example:
At 1.70 atm, a sample of gas takes up 4.25L. If the pressure in the gas is increased to 2.40 atm,
what will the new volume be?
Understanding And Applying Boyle’s Law
Example:
A sample of Ne gas occupies 0.220L at 0.86 atm. What will be its volume at 29.4kPa?
Charles' Law
Charles' Law states that the volume of a given mass of a gas is directly proportional to its Kelvin temperature at constant pressure. In mathematical terms, the relationship between temperature and volume is expressed as V1/T1=V2/T2.
What Is The Relationship Between Volume And Temperature Of A Gas
A lesson on how to solve problems using Charles' Law.
Example:
A balloon takes up 625L at 0°C. If it is heated to 80°C, what will its new volume be?
Understanding And Applying Charles' Law
Example:
A gas at 40.0°C occupies a volume of 2.32L. If the temperature is raised to 75.0°C,
what will the new volume be if the pressure is constant?
Gay-Lussac’s Law
Gay-Lussac’s Law states that the pressure of a given mass of gas varies directly with the Kelvin temperature when the volume remains constant. Gay-Lussac’s Law is expressed in a formula form as P1/T1 = P2/T2. When dealing with Gay-Lussac’s Law, the unit of the temperature should always be in Kelvin.
Using Gay-Lussac’s Law To Understand The Relationship Between A Gas' Pressure And Temperature
Example:
If the pressure in a car tire is 1.88 atm at 25°C, what will be the pressure if the temperature
warms to 37°C?
How To Solve Word Problems That Show How To Use Gay-Lussac’s Law?
Examples:
- The pressure in a sealed can of gas is 235kPA when it sits at room temperature (20°). If the can is warmed to 48°C, what will the new pressure inside the can be?
- A car tire has a pressure of 2.38 atm at 15.2°C. If the pressure inside reached 4.08 atm, the tire will explode. How hot would the tire have to get for this to happen? Report the temperature in degrees Celsius.
Practice Problem to show how to use Gay-Lussac’s Law
Example:
In the morning, a paintball pressure tank is at 306 atm. The weather heats up over the course of the
day, and by 3 PM, the outside temperature is roasting at 38.5°C, and the pressure inside the
tank is 324 atm. What was the temperature (in degree Celsius) in the morning?
Combined Gas Law
The Combined Gas Law combines Charles' Law, Boyle’s Law and Gay Lussac’s Law. The Combined Gas Law states that a gas' (pressure × volume)/temperature = constant.
Example:
A gas at 110kPa at 30.0°C fills a flexible container with an initial volume of 2.00L. If the temperature is raised to 80,0°C and the pressure increases to 440Kpa, what is the new volume?
How To Solve Problems With The Combined Gas Equation?
Example:
A 40.0L balloon is filled with air at sea level (1.00 atm, 25.0°C). It is tied to a rock and
thrown in a cold body of water, and it sinks to the point where the temperature is 4.0°C and the pressure is 11.0 atm. What will its new volume be?
Ideal Gas Law
The Ideal Gas Law mathematically relates the pressure, volume,
amount and temperature of a gas with the equation:
pressure × volume = moles × ideal gas constant × temperature;
PV = nRT.
The Ideal Gas Law is ideal because it ignores interactions between the gas particles in order to simplify the equation. There is also a Real Gas Law which is much more complicated and produces a result which, under most circumstances, is almost identical to that predicted by the Ideal Gas Law.
Understanding And Applying The Ideal Gas Law
Example:
What is the pressure in atm of a 0.108 mol sample of the gas at a temperature of 20.0°C if its
volume is 0.505L?
Sample Problems For Using The Ideal Gas Law, PV = nRT
Examples:
- 2.3 moles of Helium gas are at a pressure of 1.70 atm, and the temperature is 41°C. What is the volume of the gas?
- At a certain temperature, 3.24 moles of CO2 gas at 2.15 atm take up a colume of 35.28L. What is this temperature (in Celsius)?
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.