Types of Exponents
In this lesson, we will give a short summary of exponents: positive exponents, zero exponents, negative exponents, fractional exponents, adding exponents and multiplying exponents.
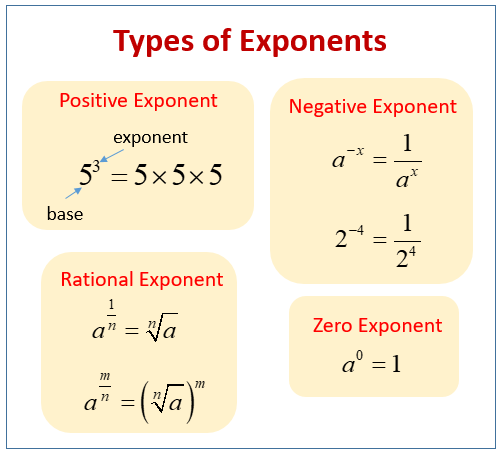
The following diagram shows the types of exponents: positive exponents, negative exponents, rational exponents, and zero exponents. Scroll down the page for more examples and solutions for the types of exponents.
Positive Exponents
Exponents are a shorthand way to show how many times a number, called the base, is multiplied to itself. A number with an exponent is said to be “raised to the power” of that exponent.
Example:
Two raised to the power of three = 23 = 2 × 2 × 2 = 8
Any number raised to the power of one equals itself, for example:
151 = 15
Zero Exponents
Any number (except 0) raised to the zero power equals 1, for example:
3220= 1
Negative Exponents
A negative exponent means that the base is changed to its reciprocal and then raised to the power of the positive exponent, for example:
5-3 = 
54 x 5-3 = 54-3 = 5
The following video show more examples of negative exponents:
Fractional Exponent
A fractional exponent in the form of 
means to take the nth root of the base, for example:
is the square root of 4.
The following video show more examples of fractional exponents:
Adding Exponents
If two numbers with exponents have the same base, you can multiply them by adding the exponents, for example:
4510 × 4531 = 4541
Multiplying Exponents
When you have a power of a power, you multiply the exponents, for example:
The Rules Of Exponents
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.