Illustrative Mathematics Unit 6.2, Lesson 14: Solving Equivalent Ratio Problems

Related Pages
Illustrative Math
Grade 6

Lesson 14: Solving Equivalent Ratio Problems

How to solve equivalent ratio problems?

Illustrative Math Unit 6.2, Lesson 14 (printable worksheets)

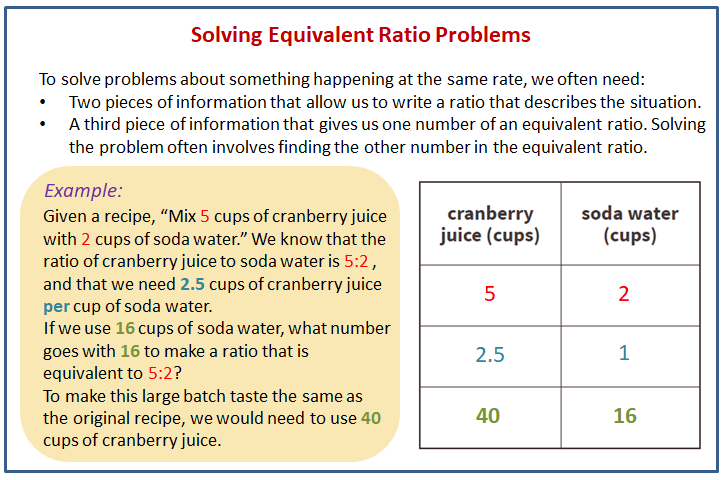
Lesson 14 Summary

The following diagram shows how to solve equivalent ratio problems.

14.1 What Do You Want to Know?

Here is a problem: A red car and a blue car enter the highway at the same time and travel at a constant speed. How far apart are they after 4 hours?
What information would you need to solve the problem?

• See Video 1 for Whole Lesson
• See Video 2 for Whole Lesson

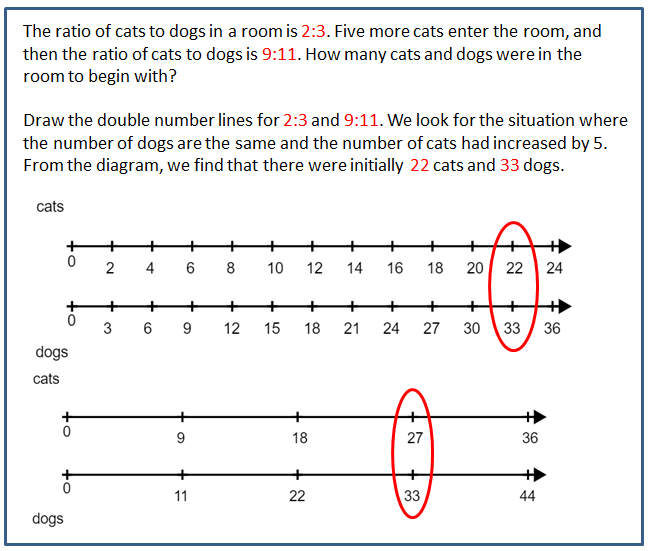
Scroll down the page for the solution to the number of cats and dogs.

14.2 - Info Gap: Hot Chocolate and Potatoes

Your teacher will give you either a problem card or a data card. Do not show or read your card to your partner.

If your teacher gives you the problem card:

1. Read your card silently and think about what you need to know to be able to answer the questions.
2. Ask your partner for the specific information that you need.
3. Explain how you are using the information to solve the problem.
4. Solve the problem and show your reasoning to your partner.

If your teacher gives you the data card:

1. Read your card silently.
2. Ask your partner “What specific information do you need?” and wait for them to ask for information.
   If your partner asks for information that is not on the card, do not do the calculations for them. Tell them you don’t have that information.
3. Have them explain “Why do you need that information?” before telling them the information.
4. After your partner solves the problem, ask them to explain their reasoning, even if you understand what they have done.
   Both you and your partner should record a solution to each problem.

14.3 - Comparing Reading Rates

• Lin read the first 54 pages from a 270-page book in the last 3 days.
• Diego read the first 100 pages from a 320-page book in the last 4 days.
• Elena read the first 160 pages from a 480-page book in the last 5 days.
  If they continue to read every day at these rates, who will finish first, second, and third? Explain or show your reasoning.

Are you ready for more?

The ratio of cats to dogs in a room is 2:3. Five more cats enter the room, and then the ratio of cats to dogs is 9:11. How many cats and dogs were in the room to begin with?

• Show Answer

  

Lesson 14 Practice Problems

1. A chef is making pickles. He needs 15 gallons of vinegar. The store sells 2 gallons of vinegar for $3.00 and allows customers to buy any amount of vinegar. Decide whether each of the following ratios correctly represents the price of vinegar.
   a. 4 gallons to $3.00
   b. 1 gallon to $1.50
   c. 30 gallons to $45.00
   d. $2.00 to 30 gallons
   e. $1.00 to 2/3 gallon
   
2. A caterer needs to buy 21 pounds of pasta to cater a wedding. At a local store, 8 pounds of pasta cost $12. How much will the caterer pay for the pasta there?
   a. Write a ratio for the given information about the cost of pasta.
   b. Would it be more helpful to write an equivalent ratio with 1 pound of pasta as one of the numbers, or with $1 as one of the numbers? Explain your reasoning, and then write that equivalent ratio.
   c. Find the answer and explain or show your reasoning.
   
3. Lin is reading a 47-page book. She read the first 20 pages in 35 minutes.
   a. If she continues to read at the same rate, will she be able to complete this book in under 1 hour?
   b. If so, how much time will she have left? If not, how much more time is needed? Explain or show your reasoning.
   
4. Diego can type 140 words in 4 minutes.
   a. At this rate, how long will it take him to type 385 words?
   b. How many words can he type in 15 minutes?
   If you get stuck, consider creating a table.
   
5. A train that travels 30 miles in 1/2 hour at a constant speed is going faster than a train that travels 20 miles in 1/2 hour at a constant speed. Explain or show why.
6. Find the surface area of the polyhedron that can be assembled from this net. Show your reasoning.
   • Show Polyhedron

     

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.