Distance Formula Examples
Related Topics:
Lesson Plans and Worksheets for Grade 8
Lesson Plans and Worksheets for all Grades
More Lessons for Grade 8
Common Core For Grade 8
Videos, examples, solutions, and lessons to help Grade 8 students learn how to apply the Pythagorean Theorem to find the distance between two points in a coordinate system.
Common Core: 8.G.8
Suggested Learning Targets
- I can recall the Pythagorean Theorem and its converse and relate it to any two distinct points on a graph.
- I can determine how to create a right triangle from two points on a coordinate graph.
- I can use the Pythagorean Theorem to solve for the distance between the two points. Apply the Pythagorean Theorem to find the distance between two points in a coordinate system 8.G.8
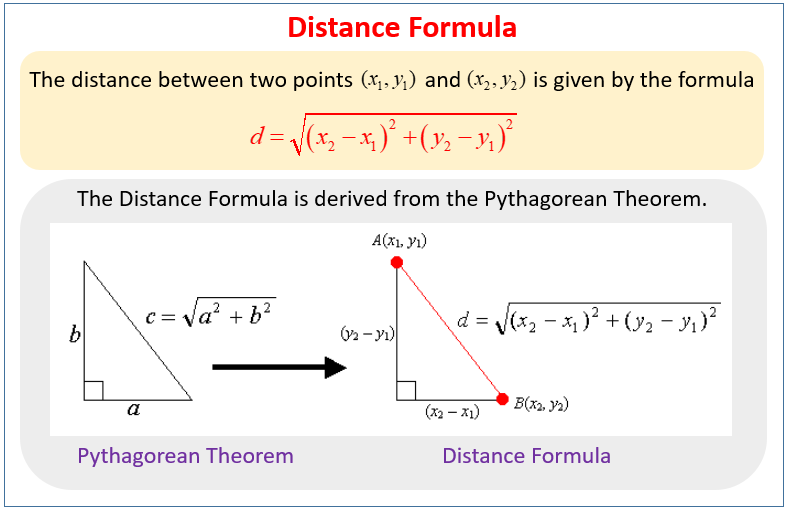
The following figures give the Distance Formula and how the Distance Formula is derived from the Pythagorean Theorem. Scroll down the page for more examples and solutions.
Distance on the Coordinate Plane 1 (8.G.8)
Using The Distance Formula Or Pythagorean Theorem To Find The Distance Between Two Points
This tutorial examines how to find the distance between two points that are shown on the coordinate plane by drawing a right triangle and using the Pythagorean Theorem to solve. Also see how to use the distance formula when only given the ordered pairs of two points.
The Distance Formula - Deriving the Formula from Pythagorean Theorem
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.