Definite Integral
The area A of the region S that lies under the graph of the continuous function f is the limit of the sum of the areas of the approximating rectangles.
Definition of a Definite Integral
Let f be a function that is continuous on the closed interval [a, b]. The definite integral of f from a and b is defined to be the limit
where
is a Riemann Sum of f [a, b]
The following diagram gives some properties of the definite integral. Scroll down the page for more examples and solutions.
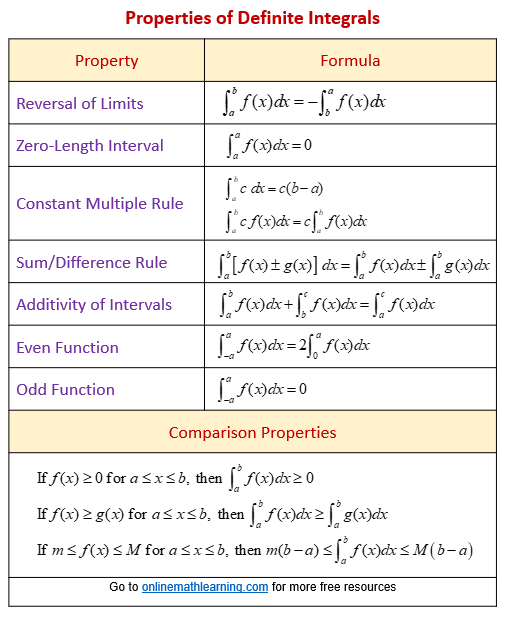
Example:
Given that ![]() , evaluate
, evaluate ![]()
Solution:

Approximating Area Using Rectangles
When finding the area under a curve for a region, it is often easiest to approximate area using a summation series. This approximation is a summation of areas of rectangles. The rectangles can be either left-handed or right-handed and, depending on the concavity, will either overestimate or underestimate the true area.
Approximating a Definite Integral Using Rectangles
This video shows how to use 4 rectangles and left endpoints as well as midpoints to approximate the area underneath 16 - x2 from x = 0 to x = 2.
The Definite Integral
The definite integral is an important operation in Calculus, which can be used to find the exact area under a curve. The definite integral takes the estimating of approximate areas of rectangles to its limit by using smaller and smaller rectangles, down to an infinitely small size.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.