Conic Sections - Circles

What is the equation of circle?

The following diagram shows how to derive the equation of circle (x - h)² + (y - k)² = r² using Pythagorean Theorem and distance formula. Scroll down the page for examples and solutions.

 

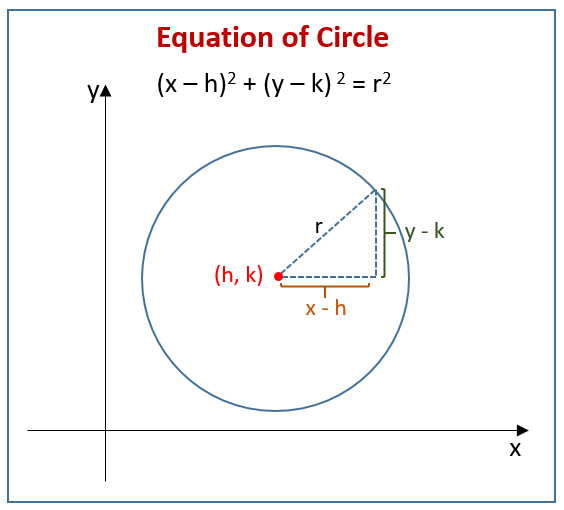
Circle Conic Section

When working with circle conic sections, we can derive the equation of a circle by using coordinates and the distance formula.
The equation of a circle is (x - h)² + (y - k)² = r² where r is equal to the radius, and the coordinates (x,y) are equal to the circle center.
The variables h and k represent horizontal or vertical shifts in the circle graph.
Examples:
1. Find the center and the radius
a) x² + (y + 2)² = 121
b) (x + 5)² + (y - 10)² = 9

2. Find the equation the circle with
a) center(-11, -8) and radius 4
b) center (2, -5) and point on circle(-7, -1)

• Show Video Lesson

How To Graph A Circle In Standard Form And General Form?

Identify the equation of a circle.
Write the standard form of a circle from general form.
Graph a circle.
A circle is the set of points (x,y) which are a fixed distance r, the radius, away from a fixed point (h,k), the center.
(x - h)² + (y - k)² = r²
Examples:
1. Graph the circle
a) (x - 3)² + (y + 2)² = 16
b) x² + (y - 1)² = 4

2. Write in standard form and then graph
2x² + 2y² - 12x + 8y - 24 = 0

• Show Video Lesson

Conic Sections

Introduction to Circles
Understand the equation of a circle

• Show Video Lesson

Graph And Write Equations Of Circles

Example:
Graph the equation
(x - 1)² + (y + 2)² = 9

• Show Video Lesson

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.