Complementary Angles
Examples, solutions, videos, worksheets, and stories to help Grade 6 students learn about complementary angles.
The following diagrams show examples of complementary and supplementary angles. Scroll down the page for more examples and step by step explanations.
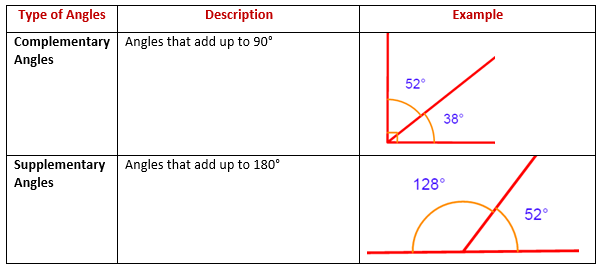
Complementary Angles
Angles in a right angle add up to 90°.
When the sum of two angles is 90° those two angles are complementary angles.
Supplementary Angles
Angles in a straight line add up to 180°
When the sum of two angles is 180° those two angles are supplementary angles.
Angles around a point
The sum of angles at a point is 360°.
Vertical Opposite Angles
When two lines intersect, two pairs of vertically opposite angles are formed.
Vertical opposite angles are equal in size.
Angle Basics
Parallel lines are lines that will never cross even if they go on forever.
When lines intersect, they form angles.
Perpendicular lines form right angles or angles that are 90°.
An angle smaller than a right angle is called an acute angle.
An angle that is greater than a right angle is called an obtuse angle.
Any two angles that form a right angle are called complementary angles.
Any two angles that form a straight line are called supplementary angle.
See the following video, If you need help understanding angles.
Complementary & Supplementary Angles
See the following video if you need help understanding complementary and supplementary angles.
It will go through complementary and supplementary angle examples and explain the steps of how to find a missing complementary angle measure and/or supplementary angle measure.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.