Illustrative Mathematics Grade 7, Unit 7, Lesson 7: Building Polygons (Part 2)

Related Pages
Illustrative Math
Grade 7

Lesson 7: Building Polygons (Part 2)

Let’s build more triangles.

Illustrative Math Unit 7.7, Lesson 7 (printable worksheets)

Lesson 7 Summary

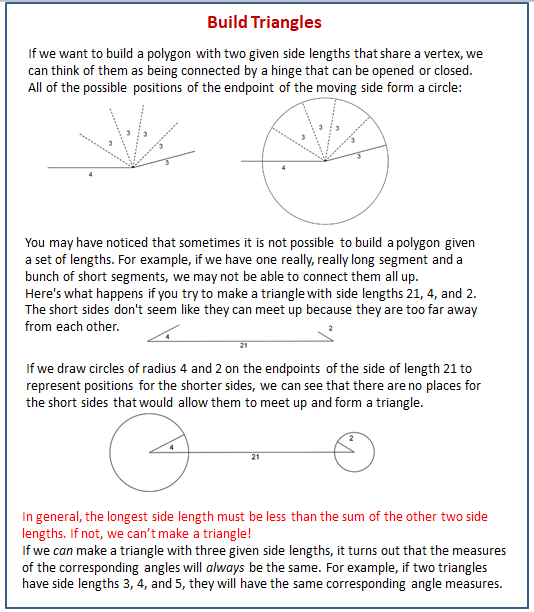
The following diagrams explain how to know whether or not 3 side lengths will make a triangle.

Lesson 7.1 Where Is Lin?

At a park, the slide is 5 meters east of the swings. Lin is standing 3 meters away from the slide.

1. Draw a diagram of the situation including a place where Lin could be.
2. How far away from the swings is Lin in your diagram?
3. Where are some other places Lin could be? Open Applet

• See Video for Whole Lesson

Lesson 7.2 How Long Is the Third Side?

Use the applet to answer the questions.

1. Build as many different triangles as you can that have one side length of 5 inches and one of 4 inches. Record the side lengths of each triangle you build.
2. Are there lengths that could be used for the third side of the triangle that aren’t values of the sliders?
3. Are there lengths that are values of the sliders that could not be used as the third side of the triangle? Open Applet

Are you ready for more?

Assuming you had access to strips of any length, and you used the 9-inch and 5-inch strips as the first two sides, complete the sentences:

1. The third side can’t be _____ inches or longer.
   • Show Answer

     9 + 5 = 14
     The third side can’t be 14 inches or longer.

2. The third side can’t be _____ inches or shorter.
   • Show Answer

     9 - 5 = 4
     The third side can’t be 4 inches or shorter.

Lesson 7.3 Swinging the Sides Around

Use the applet to answer the questions.

1. If you wanted to make a triangle with side lengths of 3 units, 3 units, and 4 units, how would you know at what angle you should position the sides?
2. Let segment AC be the 3-unit side length. Right-click on point C, check Trace On, and drag point C.
3. What path does point C trace as you move it around? Why? What other tool do we have in our geometry toolkit that could do something similar?
4. Using 2 different colors, draw 2 different triangles that would have the 4-unit segment as their base and a 3-unit segment for their right side.
5. Right-click on point , uncheck Trace On.
6. Repeat the previous instructions, letting segment BD be the 3-unit side length.
7. Using a third color, draw a point where the two traces intersect. Next, draw another triangle that would have segment AB as its base and segments AC and AD for both of its other sides.
8. If you had used segments that were only 2 units long on either end of segment , how would that have affected your drawing?

Open Applet

Lesson 7 Practice Problems

1. In the diagram, the length of segment AB is 10 units and the radius of the circle centered at A is 4 units. Use this to create two unique triangles, each with a side of length 10 and a side of length 4. Label the sides that have length 10 and 4.
2. Select all the sets of three side lengths that will make a triangle.
3. Based on signal strength, a person knows their lost phone is exactly 47 feet from the nearest cell tower. The person is currently standing 23 feet from the same cell tower. What is the closest the phone could be to the person? What is the furthest their phone could be from them?
4. Each row contains the degree measures of two complementary angles. Complete the table.
5. Here are two patterns made using identical rhombuses. Without using a protractor, determine the value of a and b. Explain or show your reasoning.
6. Mai’s family is traveling in a car at a constant speed of 65 miles per hour.
   a. At that speed, how long will it take them to travel 200 miles?
   b. How far do they travel in 25 minutes?

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.