Area of 2-D Shapes
A series of free, online High School Geometry Video Lessons.
Videos, worksheets, and activities to help Geometry students.
In this lesson, we will learn
- how to find the area of a parallelogram
- how to find the area of a triangle
- how to find the area of a trapezoid
- how to solve formulas for geometry
The following figures give the area formulas for rectangle, parallelogram, triangle, and trapezoid. Scroll down the page for more examples and solutions.
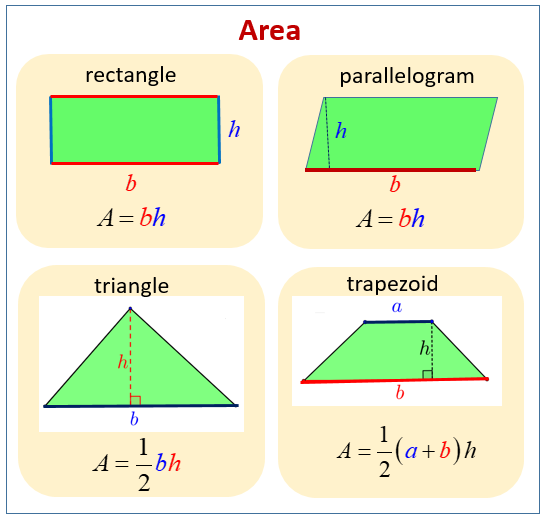
Area of Parallelograms
The area of parallelograms formula is derived from the area of a rectangle. By drawing an altitude from one vertex in a parallelogram and translating the right triangle, a rectangle is formed. Therefore, to calculate the area of a parallelogram, multiply a height by the corresponding base. The corresponding base is the side perpendicular to the height. Related topics include area of trapezoids and rhombuses.
How to find the area of any parallelogram using rectangle area formulas.
Area of Triangles
The formula for calculating the area of triangles comes from dividing a parallelogram in half, so the area is half of base times height. When finding the area of a triangle, the height is an altitude and the base must be the side intersected by the altitude. When given the area and asked for a base or height, a common mistake is to forget to multiply both sides of the equation by 2 before dividing. Area of Rectangles, Triangles and Parallelograms
How to derive the area of a triangle formula using the rectangle or parallelogram area formula.
Area of Trapezoids
The area formula for a trapezoid is found by making a parallelogram made up of two congruent trapezoids. To do this, copy a trapezoid, rotate the copy 180 degrees, and translate to create a parallelogram. The area of a parallelogram is base times corresponding height; since there are two trapezoids, the area of trapezoids formula must be divided in half. Since the bases are not congruent, they must be summed separately.
How to derive the area of a trapezoid formula using the area of a rectangle.
Visualizing Area of a Trapezoid Formula - Deriving the Formula
How to Find the Area of a Trapezoid
Solving Formulas
Solving formulas for a variable is a critical skill in the Geometry area unit because many problems will give the area of a polygon and ask for a side, height, or some other dimension. In these cases, simply substituting and typing into a calculator will not yield the correct answer. The successful Geometry student must be capable of substituting into a formula and then solving formulas for the one remaining variable.
How to strategize about solving formulas for variables; how to solve the area of a rectangle formula for base and height; how to solve the area of a triangle formula for base and for height.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.