Illustrative Mathematics Grade 7, Unit 3, Lesson 8: Relating Area to Circumference

Related Pages
Illustrative Math
Grade 7

Lesson 8: Relating Area to Circumference

Let’s rearrange circles to calculate their areas.

Illustrative Math Unit 7.3, Lesson 8 (printable worksheets)

Lesson 8 Summary

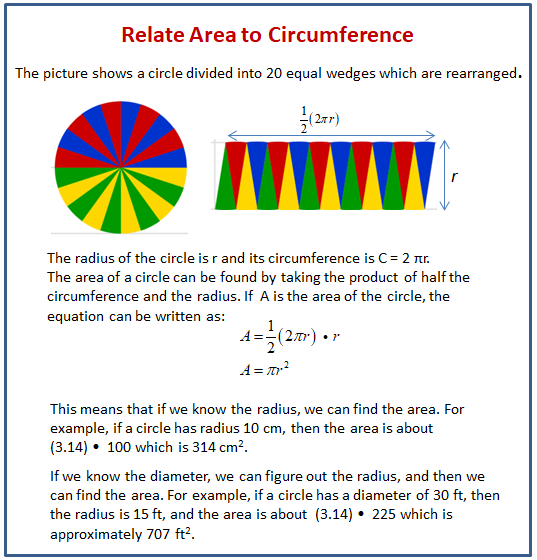
The following diagram shows how to relate the area of a circle and its circumference and find the formula for area of a circle.

Lesson 8.1 Irrigating a Field

A circular field is set into a square with an 800 m side length. Estimate the field’s area.
A. About 5,000 m²
B. About 50,000 m²
C. About 500,000 m²
D. About 5,000,000 m²
E. About 50,000,000 m²

• See Video for Whole Lesson

Lesson 8.2 Making a Polygon out of a Circle

Your teacher will give you a circular object, a marker, and two pieces of paper of different colors.
Follow these instructions to create a visual display:

1. Using a thick marker, trace your circle in two separate places on the same piece of paper.
   
2. Cut out both circles, cutting around the marker line.
   
3. Fold and cut one of the circles into fourths.
   
4. Arrange the fourths so that straight sides are next to each other, but the curved edges are alternately on top and on bottom. Pause here so your teacher can review your work.
   
5. Fold and cut the fourths in half to make eighths. Arrange the eighths next to each other, like you did with the fourths.
6. If your pieces are still large enough, repeat the previous step to make sixteenths.
   
7. Glue the remaining circle and the new shape onto a piece of paper that is a different color.
   After you finish gluing your shapes, answer the following questions.
   
8. How do the areas of the two shapes compare?
   
9. What polygon does the shape made of the circle pieces most resemble?
   
10. How could you find the area of this polygon?
    

Lesson 8.3 Making Another Polygon out of a Circle

Imagine a circle made of rings that can bend but not stretch.
Watch the animation.
Open Applet

1. What polygon does the new shape resemble?
2. How does the area of the polygon compare to the area of the circle?
3. How can you find the area of the polygon?
4. Show, in detailed steps, how you could find the area in terms of the circle’s measures. Show your thinking. Organize it so it can be followed by others.
5. After you finish, trade papers with a partner and check each other’s work. If you disagree, work to reach an agreement. Discuss:
   Do you agree or disagree with each step?
   Is there a way they can make the explanation clearer?
   
6. Revise your explanation based on your partner’s feedback.

Lesson 8.4 Tiling a Table

Elena wants to tile the top of a circular table. The diameter of the table top is 28 inches. What is its area?

Are you ready for more?

A box contains 20 square tiles that are 2 inches on each side. How many boxes of tiles will Elena need to tile the table?

• Show Answer

  Assuming that we can break up the tiles to cover the table:
  The area of the table from the previous question is 615.44 inches².
  Each tile is 2 · 2 = 4inches²
  The number of tiles required = 154 tiles (615.44 ÷ 4 = 153.86)
  Number of boxes = 8 (154 ÷ 20 = 7.7)

Lesson 8 Practice Problems

1. The picture shows a circle divided into 8 equal wedges which are rearranged.
   The radius of the circle is r and its circumference is 2πr. How does the picture help to explain why the area of the circle is πr²?
   
2. A circle’s circumference is approximately 76 cm. Estimate the radius, diameter, and area of the circle.
   
3. Jada paints a circular table that has a diameter of 37 inches. What is the area of the table?
   
4. The Carousel on the National Mall has 4 rings of horses. Kiran is riding on the inner ring, which has a radius of 9 feet. Mai is riding on the outer ring, which is 8 feet farther out from the center than the inner ring is.
   a. In one rotation of the carousel, how much farther does Mai travel than Kiran?
   b. One rotation of the carousel takes 12 seconds. How much faster does Mai travel than Kiran?
   
5. Here are the diameters of four coins:
   a. A coin rolls a distance of 33 cm in 5 rotations. Which coin is it?
   b. A quarter makes 8 rotations. How far did it roll?
   c. A dime rolls 41.8 cm. How many rotations did it make?
   

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.