

Adding and Subtracting Radicals
Videos, worksheets, solutions, and activities to help Grade 9 students learn about adding and subtracting radicals.
Related Topics:
More Lessons for Grade 9
Math Worksheets
The following diagram shows the parts of a radical: radical symbol, radicand, index, and coefficient. Scroll down the page for examples and solutions.
In order to add or subtract radicals, we must have "like radicals" that is the radicands and the index must be the same for each term. The radicand is the number inside the radical.
Radicals that are "like radicals" can be added or subtracted by adding or subtracting the coefficients.
1. Break down the given radicals and simplify each term.
2. Identify the like radicals. 3. Add or subtract the like radicals by adding or subtracting their coefficients.
Examples:
1. 4√5 + 3√5
2. -3√75 - √27
Adding and subtracting radicals
Students learn to add or subtract radicals by first breaking down the given radicals and simplifying each term, then combining terms that have the same number inside the radical.
Example:
5√18 + 3√8 - √50
Adding and Subtracting Radical Expressions
Examples:
Simplify
1. 3√6 - 4√6
2. -3√7 + 4√7
3. -11√21 + 11√21
4. -2√3 + 3√27
5. 2√6 - 2√24
6. 2√6 + 3√54
7. -√12 + 3√3
8. 3√3 - √27
9. 3√8 + 3√2
Adding & Subtracting Radical Expressions
This video looks at adding and subtracting radical expressions (square roots). It includes four examples.
Examples:
1. 2√5 + 6√5
2. √b + 6√2b - 5√b
2. 2√12 + 6√3
2. √200 + √75


Related Topics:
More Lessons for Grade 9
Math Worksheets
The following diagram shows the parts of a radical: radical symbol, radicand, index, and coefficient. Scroll down the page for examples and solutions.
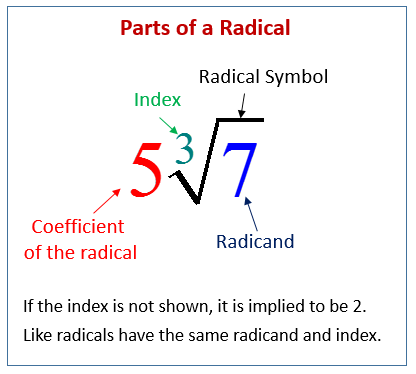
In order to add or subtract radicals, we must have "like radicals" that is the radicands and the index must be the same for each term. The radicand is the number inside the radical.
Radicals that are "like radicals" can be added or subtracted by adding or subtracting the coefficients.
1. Break down the given radicals and simplify each term.
2. Identify the like radicals. 3. Add or subtract the like radicals by adding or subtracting their coefficients.
Examples:
1. 4√5 + 3√5
2. -3√75 - √27
Adding and subtracting radicals
Students learn to add or subtract radicals by first breaking down the given radicals and simplifying each term, then combining terms that have the same number inside the radical.
Example:
5√18 + 3√8 - √50
Examples:
Simplify
1. 3√6 - 4√6
2. -3√7 + 4√7
3. -11√21 + 11√21
4. -2√3 + 3√27
5. 2√6 - 2√24
6. 2√6 + 3√54
7. -√12 + 3√3
8. 3√3 - √27
9. 3√8 + 3√2
Adding & Subtracting Radical Expressions
This video looks at adding and subtracting radical expressions (square roots). It includes four examples.
Examples:
1. 2√5 + 6√5
2. √b + 6√2b - 5√b
2. 2√12 + 6√3
2. √200 + √75
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.



We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.