Factoring Perfect Square Trinomials
In these lessons, we will learn how to factor perfect square trinomials.
Related Pages
Factoring Trinomials
Factoring Trinomials Worksheets
Algebra Lessons
Math Worksheets
The following diagrams show the factoring and expanding of Perfect Square Trinomials. Scroll down the page for examples and solutions of factoring Perfect Square Trinomials.
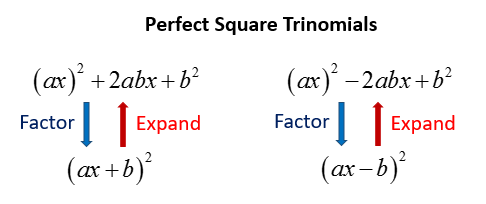
Perfect Square Trinomials
In some cases recognizing some common patterns in the trinomial will help you to factor it faster. For example, we could check whether the trinomial is a perfect square.
A perfect square trinomial is of the form:
(ax)2 + 2abx + b2
Take note that:
- The first term and the last term are perfect squares.
- The coefficient of the middle term is twice the square root of the last term multiplied by the square root of the coefficient of the first term.
When we factor a perfect square trinomial, we will get.
(ax)2 + 2abx + b2 = (ax + b)2
The perfect square trinomial can also be in the form:
(ax)2 – 2abx + b2,
in which case it will factor as follows:
(ax)2 – 2abx + b2 = (ax <– b)2
Example:
Factor the following trinomials:
a) x2 + 8x + 16
b) 4x2– 20x + 25
Solution:
a) x2 + 8x + 16
= x2 + 2(x)(4) + 42
= (x + 4)2
b) 4x2– 20x + 25
= (2x)2– 2(2x)(5) + 52
= (2x – 5)2
Example:
Factor and solve the following equations:
a) x2 + 2x + 1 = 0
b) x2 + 6x + 9 = 0
Solution:
a)x2 + 2x + 1 = 0
⇒ (x + 1)2 = 0
⇒ x + 1 = 0
⇒ x = − 1
b) x2 + 6x + 9 = 0
⇒ x2 + 2(3)x + 32 = 0
⇒ (x + 3)2 = 0
⇒ x + 3 = 0
⇒ x = − 3
How To Factor A Perfect Square Trinomial?
One special case when trying to factor polynomials is a perfect square trinomial. Unlike a difference of perfect squares, perfect square trinomials are the result of squaring a binomial. It’s important to recognize the form of perfect square trinomials so that we can easily factor them without going through the steps of factoring trinomials, which can be very time consuming.
To factor a perfect square trinomial we need to be able to recognize perfect square factors.
a2 + 2ab + b2 = (a + b)(a + b) = (a + b)2
a2 - 2ab + b2 = (a - b)(a - b) = (a - b)2
Example:
Factoring
4x2 - 12x + 9
16x2 + 56x + 49
Example:
Factor 9x2 + 24xy + 16y2
Example:
Factor 81x2 − 36xy + 4y2
How To Identify And Factor Perfect Square Trinomials?
- The sign between the terms are positive or different.
- If you double the root of the first and last terms you get the middle term.
Example:
Determine if the given polynomial is a perfect square trinomial. If it is, factor and check your answer.
- 4x2 - 20xy + y2
- 9x2 + 12x + 4
Example:
Factor (2p + t)2 + 6(2p + t) + 9
Example:
This video shows how to factor the following perfect square trinomials.
v2 + 14v + 49
t2 + 1/3t + 1/36
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.